Information
AI Chat

PEM 2020 Mathematics Advanced Preliminary Exam

PEM Exam Y11

Subject

math advanced

513 Documents

Students shared 513 documents in this course

Degree • Grade

Minarah College

Academic year: 2023/2024

Listed booksC++Fundamentals of Actuarial Mathematics Plancyclus in het Sociaal-Agogisch Werk What is Cinema?Anne Peters

Uploaded by:

Nour Wehbe

Minarah College

0followers

3Uploads

0upvotes

Recommended for you

14
Past HSC - Trig Ratios, Sine and Cosine Rules to 2022
math advanced
Practice materials
100%(3)
7
C1 Tangents - Math Revision for Year 11 Maths Adv; Topic Specific
math advanced
Practice materials
100%(2)
14
Bearing - Math Revision for Year 11 Maths Adv; Topic Specific
math advanced
Practice materials
100%(2)
19
ACE 2020 Year 11 Mathematics Advanced
math advanced
Practice materials
100%(1)
10
E1 Logs and Index Laws and Equations
math advanced
Practice materials
100%(1)

Comments

Please sign in or register to post comments.

Related Studylists

math

Preview text

Student Number

Mathematics Advanced

General Instructions

Reading time – 5 minutes Total Marks – 70
Working time – 2 hours
Write using blue or black pen Section I Questions 1 – 10 10 marks
Board–approved calculators may be Allow about 15 minutes for this section

used

A reference sheet is provided at Section II Questions 11 – 32 6 0marks

the back of this paper Allow about 1 hour and 45 minutes for

All necessary working should be this section

shown in Questions 11 – 32

2020

PRELIMINARY EXAMINATION

Directions to School or College To ensure integrity and security, examination papers must NOT be removed from the examination room. Examination papers may not be returned to students until 24th September, 2020. These examination papers are supplied Copyright Free, as such the purchaser may photocopy and/or make changes for educational purposes within the confines of the School or College. All care has been taken to ensure that this examination paper is error free and that it follows the style, format and material content in accordance with the NESA requirements, recommendations and guidelines. No guarantee or warranty is made or implied that this examination paper mirrors in every respect alternative Preliminary Examination papers written for this course.

Section I

10 marks

Attempt Questions 1- 10

Allow about 15 minutes for this section

Use the multiple-choice answer sheet for Questions 1-10.

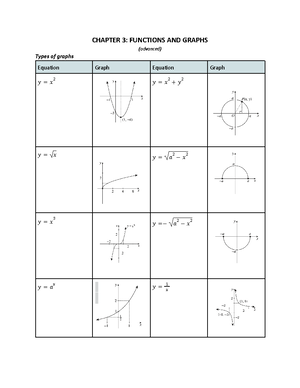
Which graph does not represent a function?

(A) (B)

(C) (D)

What is the greatest value for f ( )x = 5 −2sinx?

(A) − 3

(B) 3

(C) 5

(D) 7

The points A and P are on the unit circle

2 2 x + y = 1. A(1, 0)and O is the origin.

What are the exact coordinates of the point P, if AOP = 120?

(A)

1 1

,

2  

 − 

 

(B)

1 1

,

2 2

 

−

 

 

(C)

1 3

,

2 2

 

 − 

 

 

(D)

3 1

,

2 2

 

 − 

 

 

In a raffle, 100 tickets are sold and 3 prizes are given away. A person buys 5 tickets.

What is the probability that this person wins at least one prize?

(A)

1

20 (B)

893 19404

(C)

4657

32340

(D)

893 6468

Which of the following graphs shows the function 2 y = −x x ( − 1) ( x+1)?

(A) (B)

(C) (D)

Section II

60 marks

Attempt Questions 11- 32

Allow about 1 hours and 45 minutes for this section

Answer the questions in the spaces provided. Sufficient spaces are provided

for typical responses.

Your responses should include relevant mathematical reasoning and/or calculations.
Extra writing space is provided at the back of this booklet on pages 26 and 27.

If you use this space, clearly indicate which question you are answering.

Question 11 (1 mark)

Solve 5 − 2 x 17 1

..................................................................

Question 12 (1 mark)

Express as a single fraction in its most simplest form

3 2

2 3

x − x+ −. 1

..................................................................

Question 13 (3 marks)

Consider f ( )x = 2 x− 1

(a) Sketch f ( )x showing all intercepts and features. 1

............................................................

(b) Hence, or otherwise, solve f ( )x = 5. 2

............................................................

Question 16 (3 marks)

Differentiate the following:

(a)

3 2

y = 3 x − 10 x + 1 1

............................................................

(b)

3 y = 5 −x 2

............................................................

Question 17 (2 marks)

Find the equation of the tangent to

2 1

y = e + at the point (0,3). 2

..................................................................

Question 18 (2 marks)

Find the range of values of k for which the expression ( )

2 x − 4 x + 2 +k is 2

always positive.

..................................................................

Question 19 (4 marks)

The amount of ice-cream (M) in mL that is melting can be modelled by the

function

2 M = 350 − 3 t − 5 t, where tis in minutes. Find:

(a) The amount of ice-cream initially. 1

............................................................

(b) The time it takes for the ice-cream to completely melt. 2

............................................................

Question 21 (3 marks)

Bucket 1 contains 6 green balls and 4 blue balls. Bucket 2 contains 5 green balls

and 3 red balls. A ball is drawn at random from Bucket 1 and then placed into Bucket 2.

(a) If a ball is drawn from random from the 9 balls in Bucket 2, 1

what is the probability it is green?

............................................................

(b) Given the ball from Bucket 2 is green, what is the probability 2

that the ball transferred from Bucket 1 was blue?

............................................................

Question 22 (3 marks)

A helicopter leaves a helipad at point A on a bearing of 128 for 3 to a

checkpoint at C. It then travels on a bearing of 066 for 4 to its base at B.

(a) What is the size of angle ACB? 1

............................................................

(b) What is the distance from the helipad (A) to the base (B) 2

to one decimal place?

............................................................

Question 24 (2 marks)

Find the value of p such that

2 k x ( ) = ( x − 2) +px is an even function. 2

..................................................................

Question 25 (2 marks)

Solve 2log 5 7 = log 2 7 x−log 3 7. 2

..................................................................

Question 26 ( 5 marks)

Let

( ) for - 1 co

sin

x f x x x

=   

−

.

(a) Prove that

1 cos 1 cos

sin x x x

= +

−

. 2

............................................................

(b) State any value(s) of x for which f ( )x is undefined in the given domain. 1

............................................................

( ) for - 1 co

sin

x f x x x

=   

−

. 2

............................................................

Question 28 (2 marks)

The senior group of a high school contains 100 students. Each student studies 2

at least one of the subjects A, B, C. The diagram below shows the number of

students studying each subject. The probability a student studies exactly 2

subjects is 28%. Find x and y.

..................................................................

Multiple Choice

Multiple Choice

Was this document helpful?

PEM 2020 Mathematics Advanced Preliminary Exam

Subject: math advanced

513 Documents

Students shared 513 documents in this course

Degree • Grade:

HSC

• 11

Multiple Choice

Multiple Choice

Was this document helpful?

- 1 -

Student Number

Mathematics Advanced

General Instructions

• Reading time – 5 minutes Total Marks – 70

• Working time –

hours

• Write using blue or black pen Section I Questions 1 – 10 10marks

• Board–approved calculators may be Allow about 15 minutes for this section

used

• A reference sheet is provided at Section II Questions 11 – 32 60marks

the back of this paper Allow about 1 hour and 45 minutes for

• All necessary working should be this section

shown in Questions 11 – 32

FULL NAME:__________________________

STUDENT NUMBER:_____________

2020

PRELIMINARY EXAMINATION

Directions to School or College

To ensure integrity and security, examination papers must NOT be removed from the examination room. Examination papers may

not be returned to students until 24th September, 2020. These examination papers are supplied Copyright Free, as such the

purchaser may photocopy and/or make changes for educational purposes within the confines of the School or College.

All care has been taken to ensure that this examination paper is error free and that it follows the style, format and material content

in accordance with the NESA requirements, recommendations and guidelines. No guarantee or warranty is made or implied that this

examination paper mirrors in every respect alternative Preliminary Examination papers written for this course.

PEM 2020 Mathematics Advanced Preliminary Exam

math advanced

HSC

Minarah College

Recommended for you

Comments

Students also viewed

Related documents

Related Studylists

Preview text

Mathematics Advanced

General Instructions

2020

PRELIMINARY EXAMINATION

(A) (B)

(C) (D)

(A) − 3

(B) 3

(C) 5

(D) 7

(A)

1 1

,

2

 

 − 

 

(B)

1 1

,

2 2

 

−

 

 

(C)

1 3

,

2 2

 

 − 

 

 

(D)

3 1

,

2 2

 

 − 

 

 

(A)

1

20

(B)

893

19404

(C)

4657

32340

(D)

893

6468

(A) (B)

(C) (D)

..................................................................

..................................................................

..................................................................

..................................................................

..................................................................

3 2

2 3

..................................................................

..................................................................

..................................................................

..................................................................

..................................................................

..................................................................

..................................................................

..................................................................