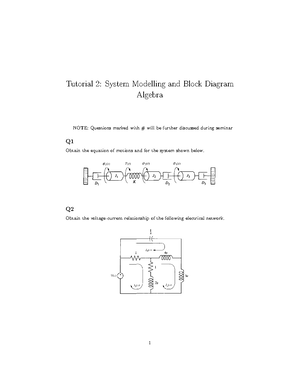
- Information
- AI Chat
Was this document helpful?
ES2C6 Week 6 Examples - qwcdwq
Module: Electromechanical System Design (ES2C6)
28 Documents
Students shared 28 documents in this course
University: The University of Warwick
Was this document helpful?

1
ES2C6 Week 6 Examples: Electromagnetics
and Motors
Lecture 6.1: Relating Current, Magnetism and Motion
Question 1: The diagram below shows a current carrying wire suspended in free space. The current
is 10A, and the permeability of free space is 𝜇0= 4𝜋 × 10−7 𝐻 𝑚
⁄. State: the direction of the
current (into or out of the page), the direction of the flux lines (clockwise or anticlockwise) and the
magnitude of the B field (flux density) at 10 mm and 1000 mm from the wire:
Solution 1: There is a cross in the wire, telling us that current flows into the page. Using the right
hand grip rule, we can see that the direction of the field is clockwise.
The equation for the magnitude of the flux density is:
0
2
I
B
r
=
We are told the current, the permeability and are given two distances:
( )
( )
7
4
10mm 3
7
6
1000mm
4 10 10 2 10 T
2 10 10
4 10 10 2 10 T
21
r
r
B
B
−
−
=−
−
−
=
==
==
You can see the magnetic flux density decreasing the further away from the wire we get.
Question 2: The diagram below shows a current carrying wire suspended in free space. The current
is 0.15𝐴, and the permeability of free space is 𝜇0= 4𝜋 × 10−7 𝐻 𝑚
⁄. State: the direction of the
current (into or out of the page), the direction of the flux lines (clockwise or anticlockwise) and the
magnitude of the B field (flux density) at 1 mm and 10 mm from the wire: