- Information
- AI Chat
Friday Solution Sheet - ed Ed FEQWEC
Electromechanical System Design (ES2C6)
The University of Warwick
Preview text
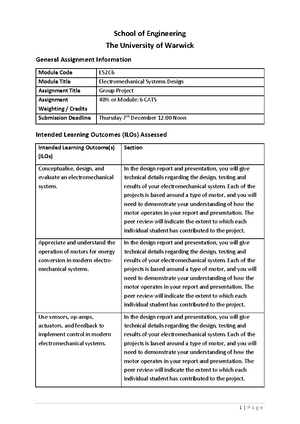
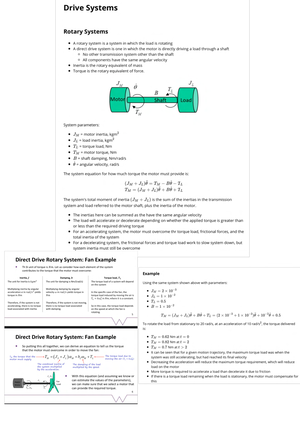
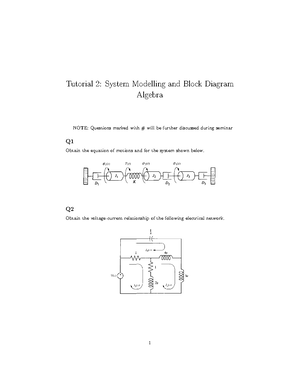
ES2C6 Electromechanical Systems Design
Week 9 Seminar: Friday Session
These questions are designed to prepare you for the QMP exam. They are derived from the material
taught in week 8: Electrical Power 1
Question 1: At time 𝑡 0 , the voltage across a 82𝑛𝐹 capacitor is 1𝑉. A constant current of 0𝑚𝐴
flows through the capacitor for 5𝑠. Calculate the voltage across the capacitor and the charge stored
in it at t = 5𝑠, and the energy stored after t = 5𝑠.
Step 1: Write down the equation to find the voltage across the capacitor:
( ) ( )
0
0 0
1 t
vC t tiC t dt V t t
C
= +
Step 2: Solve the equation using the values given in the question
( ) ( )
( )
5
9
3
9
1
0 10 1.
82 10
0 10
5 1 610 V
82 10
C
C
v t dt
v t
−
−
−
−
= +
= + =
Step 3: Calculate the charge stored in the capacitor
9
5
82 10 610.
5 10 C
Q CV
−
−
=
=
=
Step 4: Calculate the energy stored in the capacitor
( ) ( )
( ) ( ) ( )
( )
2
9 2
1
J
2
1 82
10 610.
2
15 mJ
C C
C
C
W t Cv t
W t
W t
−
=
=
=
Question 2: At time 𝑡 0 the current through a 5𝐻 inductor is 100𝑚𝐴. A constant voltage of 9𝑉 is connected across the inductor. What is the current through the inductor and energy stored in the inductor after 10𝑠? Step 1: Write down equation to find the current through inductor is:
( ) ( )
0 0 1 t iL t tvL t dt I L
= +
Step 2: Solve the equation using the values given in the question.
( )
( )
10 0 3 1 9 100 10 5 10 9 100 10 18 A 5 L L i t dt i t − − = + = + =
Step 3: Calculate the energy stored in the inductor
( ) ( )
( ) ( )( )
2 2 1 J 2 1 5. 18 J 2 819 J L L L W t Li t W t = = =
Question 4: A 50𝐻𝑧 AC sinusoidal current is given as: 𝐼(𝑡) = 240 cos(2𝜋50𝑡 − 10°) 𝐴. Express this current in the following forms:
I = A ( cos + j sin ), I = A and I = Re +jIm
Step 1: Read off the amplitude and the angle of the current A = 240 A = − 10 Step 2: Calculate the real and imaginary part of the current
( )
( ( ) ( ))
cos sin 240 cos 10 sin 10 236 41 A I A j j j = + = − + − = − Step 3: Express the current in the required terms
240 cos ( ( 10 ) sin ( 10 ))A
240 10 A 236 41 A I j I I j = − + − = − = −
Question 5: Determine the equivalent impedance in the 50Hz circuit shown below. Express the impedance in the form: Z = Re + jIm AC R L C
####### R = 10 L = 3 H C= 1 F
Step 1: Redraw the circuit in terms of impedances: AC Z1 Z2 Z Step 2: Express the impedances in phasor form 1 2 3 6 10 2 2 50 3 942. 3183. 2 2 50 1 10 Z R Z j fL j j j j Z j fC − = = = = = − − = = = − Step 3: Calculate the total impedance ZT = Z 1 + Z 2 +Z 3 1 2 3 10 942 3183. 10 2240. Z T Z Z Z j j j = + + = + − = −
Question 7: A complex load in an AC power system dissipates a complex power of 𝑆 = 1200 + 𝑗 VA. State the active (real) power, reactive power, apparent power, load angle, and sketch the power triangle. What is the power factor of the load? Step 1: We can read the real and reactive powers off the given complex power:
( )
( )
Re 1200 W Im 300 VAR P S Q S = = = = Step 2: By finding the amplitude and angle of the given complex power, we find the apparent power and load angle: 2 2 1 1200 300 1236 VA 300 tan 14. 1200 S
#######
− = + = = = Step 3: Use the load angle to find the power factor
Power Factor =cos ( )
Power Factor = cos 14 ( )=0 Lagging
Step 4: Sketch the power triangle using the values that have been calculated. P = 1200 W 14° Q = 300 VAR
Question 8: A load impedance dissipates 𝑆 = 5000∠ − 5° 𝑉𝐴. Find 𝑃, 𝑄, |𝑆|, the load angle 𝜑, the power factor and sketch the power triangle. Step 1: We can read the apparent power and the load angle off the given complex power: 5000 VA 5 S
#######
= = − Step 2: With the load angle, we can find the power factor:
Power Factor =cos ( )
Power Factor = cos ( − 5 )=0 Leading
Step 3: Find the real and imaginary parts of the complex power
( )
( ( ) ( ))
cos sin 5000 cos 5 sin 5 4981 435 VA 4981 W 435 VAR S S j j j P Q
####### = +
= − + − = − = = − Step 4: Sketch the power triangle using the values that have been calculated. P = 4981 W Q = -435 VAR 5° End of Questions Mark Dooner, 2022
Friday Solution Sheet - ed Ed FEQWEC
Module: Electromechanical System Design (ES2C6)
University: The University of Warwick
- Discover more from:
