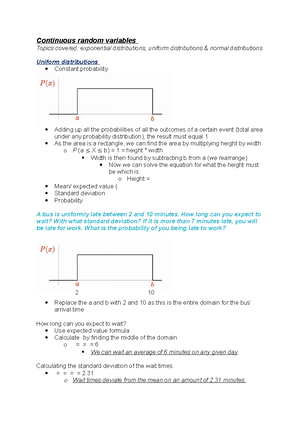
- Information
- AI Chat
Was this document helpful?
Statistics Coursework Report
Module: Statistics for business I (MA20228)
3 Documents
Students shared 3 documents in this course
University: University of Bath
Was this document helpful?

Statistics Coursework Report
Problem 1
The first thing I did for problem 1 was creating my sample using the Data Analysis: Random Number
Generation tool. I used the following criteria:
As the sample size (n) is 45, that is the amount of random numbers I requested. I gathered the
information about the distribution, p-value and number of trials from Population B.
For part a:
This is a one sample, two tailed hypothesis test with a known variance.
We know the variance as the binomial distribution states the variance for a binomial distribution is
npq, we know that the population n is 200, p is 0.6, making q 0.4 (1-0.6) – giving us 48 for our
variance, and 6.93 as our standard deviation. We can also use the binomial distribution rule to find
the mean, with the formula np, giving us a population mean of 120.
We would expect that our population mean/variance, and our sample mean/variance are similar due
to central limit theorem – and this shows true. I calculated the sample mean using the ‘average’
function in excel, the sample standard deviation (s) using the ‘st.dev’ function, and the sample
variance () by squaring the sample standard deviation.
As the number of trials in the population is 200, if the coin was fair there would be a 0.5 chance of
heads or tails. As this is a binomial experiment, we can calculate the hypothesised mean as np,
where n is 200 and p is 0.5, giving us an answer of 100. Therefore, my hypotheses are:
H0:
H1:
This is a two tailed test because we aren’t stating whether the coin is biased towards heads or tails –
just that it is biased. The significance level I will be using is 0.01 (1%).
For this question I can use the Z-Test, this is justified by the sample size being greater than 30, and
the fact we know the population variance.
The formula for finding a z-score from a sample is: . My formula is:
Using the norm.s.inv we can find the critical value for this test. Norm.s.inv gives the inverse of the
standard normal distribution (z distribution) and returns the critical value if I correctly input the
information (Cosmosweb, 2022). As it is two tailed, the alpha of 0.01 must be divided by 2 in the
Students also viewed
- Notes - Question regarding leaving a partnership or terminating a partnership
- MA20225-sheet 6-2022 - help with exercise sheets for the given lecture notes and problems
- MA20225-sheet 5-2022 - help with exercise sheets for the given lecture notes and problems
- MA20225-sheet 4-2022 - help with exercise sheets for the given lecture notes and problems
- Heart Development and Defects
- Acquired Heart Disease