Information
AI Chat

Eurocode Load combinations for steel structures

Module

Digital Structural Analysis and Design (CE32005)

12 Documents

Students shared 12 documents in this course

University

University of Dundee

Academic year: 2020/2021

Uploaded by:

hamdi zullah

University of Dundee

0followers

278Uploads

71upvotes

Comments

Please sign in or register to post comments.

Related Studylists

Steel

Preview text

A single copy of this document is licensed to

This is an uncontrolled copy. Ensure use of the

most current version of the document by searching

the Construction Information Service.

unidundee

15/02/

BCSA Publication No. 53/

Eurocode Load

Combinations

for Steel Structures

Apart from any fair dealing for the purpose of research or private study or criticism or review, as permitted under the Copyright Design and Patents Act 1988, this publication may not be reproduced, stored or transmitted in any form by any means without the prior permission of the publishers or in the case of reprographic reproduction only in accordance with the terms of the licences issued by the UK Copyright Licensing Agency, or in accordance with the terms of licences issued by the appropriate Reproduction Rights Organisation outside the UK.

Enquiries concerning reproduction outside the terms stated here should be sent to the publishers, The British Constructional Steelwork Association Ltd. at the address given below.

Although care has been taken to ensure, to the best of our knowledge, that all data and information contained herein are accurate to the extent that they relate to either matters of fact or accepted practice or matters of opinion at the time of publication, The British Constructional Steelwork Association Limited, the authors and the reviewers assume no responsibility for any errors in or misinterpretations of such data and/or information of any loss or damages arising or related to their use.

Publications supplied to members of the BCSA at a discount are not for resale by them.

The British Constructional Steelwork Association Ltd. 4, Whitehall Court, Westminster, London SW1A 2ES Telephone: +44(0)20 7839 8566 Fax: +44(0)20 7976 1634 Email: postroom@steelconstruction Website: steelconstruction

Publication Number 53/ First Edition December 2010 Second Edition July 2016

ISBN-10 1-85073-063- ISBN-13 978-1-85073-063- British Library Cataloguing-in-Publication Data A catalogue record for this book is available from the British Library © The British Constructional Steelwork Association Ltd

The British Constructional Steelwork Association Limited (BCSA) is the national organisation for the steel construction industry: its Member companies undertake the design, fabrication and erection of steelwork for all forms of construction in building and civil engineering. Industry Members are those principal companies involved in the direct supply to all or some Members of components, materials or products. Corporate Members are clients, professional offices, educational establishments etc., which support the development of national specifications, quality, fabrication and erection techniques, overall industry efficiency and good practice.

The principal objectives of the Association are to promote the use of structural steelwork; to assist specifiers and clients; to ensure that the capabilities and activities of the industry are widely understood and to provide members with professional services in technical, commercial, contractual, quality assurance and health and safety matters. The Association’s aim is to influence the trading environment in which member companies have to operate in order to improve their profitability.

A current list of members and a list of current publications and further membership details can be obtained from:

The British Constructional Steelwork Association Limited 4, Whitehall Court, Westminster, London SW1A 2ES Tel: +44(0)20 7839 8566, Fax: +44(0)20 7976 1634 Email: postroom@steelconstruction Website: steelconstruction

The British Constructional

Steelwork Association Limited

One of the most challenging aspects of the Eurocodes is gaining a thorough understanding of the loading and load combination for practical buildings. This challenge is not technical but primarily one related to the way the information is presented and the terminology used in the Eurocodes. The presentation and terminology used in the Eurocodes are very different to that found in British Standards such as BS 5950. The Eurocodes have a preference for mathematical formulae over tables and graphs and some of the explanations are brief.

The principal aim of this publication is to provide the reader with straightforward guidance on the loading and load combinations for both the serviceability and ultimate limit states for the following building types:

Multi-storey buildings – Simple construction
Multi-storey buildings – Continuous construction
Portal frames without cranes
Portal frames with cranes

Chapter 1 gives a brief introduction to EN 1990 Basis of design and EN 1991 Actions on structures together with simple explanations of the design situations presented in EN 1990. Chapter 2 is a list of abbreviations, definitions and symbols and again simple, easy to understand explanations are given. Chapter 3 gives a comprehensive description of the load combinations for both the Ultimate and Serviceability Limit States, together with a list of the load combination factors which are used to account for the reduced probability of the simultaneous occurrence of two or more variable loads. These values are based on the recommendations given in the UK National Annex for EN 1990.

Chapter 4 sets out the load combinations for both simple and moment resisting frames. Information is given on the sway sensitivity of frames, frame imperfections and the use of the equivalent horizontal force (EHF) (a general approach that replaces imperfections with a system of notional horizontal forces). Reduction factors for the number of storeys and floor area are also described together with pattern loading and overturning. Section 4 concentrates on the load combinations for simple construction while section 4 identifies the differences between simple and continuous construction. Chapter 4 concludes with a worked example that illustrates the application of the load combinations equations given in EN 1990 for a three storey high, simple braced frame.

Chapter 5 sets out the application of EN 1990 to industrial buildings with and without crane loads and illustrates the approach with the following examples:

Serviceability Limit State – Single span portal frame
Ultimate Limit State – Single span portal frame
Serviceability Limit State – Single span portal frame with overhead crane
Ultimate Limit State – Single span portal frame with overhead crane

Chapter 6 is a list of references where further guidance on applying the Eurocodes to steel and composite structures is given.

It is intended to update this publication and BCSA would appreciate any observations, particularly on inaccuracies and ambiguities, or proposals on alternative approaches or on any other matters which should be included in future editions.

This publication was prepared by: Prof. L. Gardner Imperial College London Mr. P. J. Grubb Consultant

Foreword

1. INTRODUCTION.
- 1 Background.
- 1 Introduction to EN 1990.
- 1 Introduction to EN 1991.
2. ABBREVIATIONS, DEFINITIONS AND SYMBOLS.
- 2 Abbreviations.
- 2 Definitions.
- 2 Symbols (Greek letters).
3. COMBINATIONS OF ACTIONS.
- 3 Ultimate limit states.
- 3 Serviceability limit states.
4. MULTI-STOREY BUILDINGS.
- 4 General.
- 4.1 Classification of frames.
- 4.1 Frame imperfections and equivalent horizontal forces (EHF).
- 4.1 Second order P-∆ effects.
- 4.1 Reduction factors for number of storeys ( α n) and floor area ( α A)
- 4.1 Pattern loading.
- 4.1 Dead loads.
- 4.1 Overturning.
- 4 Moment resisting frames ( continuous construction).
- 4.2 ULS load combinations based on Equation 6 with α cr> 10.
- 4.2 ULS load combinations based on Equation 6 with α cr< 10.
- 4.2 ULS load combinations based on Equation 6 & 6 with α cr> 10.
- 4.2 ULS load combinations based on Equation 6 & 6 with α cr< 10.
- 4.2 SLS load combinations.
- 4 Braced frames (simple construction).
- 4.3 ULS load combinations based on Equation 6.
- 4.3 ULS load combinations based on Equation 6 and 6.
- 4.3 SLS load combinations.
- 4 Example.
5. INDUSTRIAL BUILDINGS.
- 5 General.
- 5.1 EN 1991-1-3: 2003 - Snow loading.
- 5.1 EN 1991-1-4: 2003 - Wind loading.
- 5.1 Frame imperfections and second order P-∆ effects.
- 5 Portal frames.
- 5.2 Serviceability limit state design.
- 5.2 SLS design example for a single span portal.
- 5.2 Ultimate limit state design (STR).
- 5.2 ULS design example for a single span portal .2
- 5 Portal frames with cranes.
- 5.3 Serviceability limit state design.
- 5.3 SLS design example for a single span portal with overhead crane.
- 5.3 Ultimate limit state design (STR).
- 5.3 ULS design example for a single span portal with overhead crane.
6. REFERENCES.

1 Background.

Implementation of the structural Eurocodes is underway. The primary challenges are perceived to be related not to the technical content, but rather to the presentation and terminology of the documents, since this is very different to that found in existing UK structural design codes. Immediate differences may be observed in the preference for mathematical formulae over tables and graphs, brevity of explanations and axis conventions. The intention of this guide is to provide straightforward guidance on combinations of actions (load combinations) for the two principal types of steel structure – multi-storey buildings and industrial buildings. Further guidance on applying the Eurocodes to steel and composite structures is given in [1], [2], [3].

Each Eurocode document is accompanied by a National Annex. The National Annex contains nationally determined parameters (NDPs), which are values left open by the Eurocode for definition by the country in which the building is to be constructed.

Equation numbers employed in this guide, unless prefixed by the letter D, follow the equation numbering of EN 1990.

1 Introduction to EN 1990.

EN 1990: Eurocode – Basis of structural design is the primary Eurocode document in that it establishes the common principles and requirements that apply to all aspects of structural design to the Eurocodes. Combinations of actions for all structures are set out in EN 1990. This section provides a brief introduction to the code.

EN 1990 considers ultimate and serviceability limit states, the former being associated with the safety of people and the structure, while the latter concerns the functioning and appearance of the structure and the comfort of people. For ultimate limit states, checks should be carried out for the following, as relevant:

EQU: Loss of static equilibrium of the structure or any part of the structure.
STR: Internal failure or excessive deformation of the structure or structural members.
GEO: Failure or excessive deformation of the ground.
FAT: Fatigue failure of the structure or structural members.

In the context of structural steelwork in buildings, EQU (to assess overturning and sliding as a rigid body) and STR (to determine forces and moments in structural members under various load combinations) are of primary concern.

EN 1990 also emphasises, in Section 3, that all relevant design situations must be examined. Design situations are classified as follows, the first two being the ‘fundamental’ ones:

Persistent design situations, which refer to conditions of normal use.
Transient design situations, which refer to temporary conditions, such as during execution (construction) or repair.
Accidental design situations, which refer to exceptional conditions such as fire, explosion or impact.
Seismic design situations, which refer to conditions where the structure is subjected to seismic events.

In Clause 4.1(1) of EN 1990, actions (imposed loads and deformations) are classified by their variation with time, as permanent, variable or accidental. Permanent actions ( G ) are those that essentially do not vary with time, such as the self-weight of a structure and fixed equipment; these have generally been referred to as dead loads in previous British Standards. Variable actions ( Q ) are those that can vary with time, such as imposed loads, wind loads and snow loads; these have generally been referred to as live loads in previous British Standards. Accidental actions ( A ) are usually of short duration, but high magnitude, such as explosions and impacts. Classification by variation with time is important for the establishment of combinations of actions.

1 Introduction to EN 1991.

EN 1991 Eurocode 1 – Actions on structures comprises four parts, as given in Table 1. EN 1991-2 and EN 1991-4 are not relevant to this publication.

Table 1: Parts of EN 1991

EN 1991 Part Action type EN 1991-1 General actions EN 1991-2 Traffic loads on bridges EN 1991-3 Actions induced by cranes and machinery EN 1991-4 Silos and tanks

EN 1991-1 is sub-divided into seven sub-parts, which provide designers with most of the information required to determine each individual action on a structure. The seven sub-parts are given in Table 1, with EN 1991-1-1, EN 1991-1-3, EN 1991-1-4 and EN 1991-1-7 being of particular relevance to this publication.

Table 1: Sub-parts of EN 1991-

EN 1991-1 Part Action type EN 1991-1-1 Densities, self weight and imposed loads EN 1991-1-2 Actions on structures exposed to fire EN 1991-1-3 Snow loads EN 1991-1-4 Wind actions EN 1991-1-5 Thermal actions EN 1991-1-6 Actions during execution (construction) EN 1991-1-7 Accidental actions (impact and explosions)

EN 1991-1-1 is similar to BS 6399-1 and, since most structural designers are familiar with this document, the change to EN 1991- 1-1 will be relatively straightforward.

EN 1991-1-3 is used to determine snow loads and, although some of the terminology is unfamiliar, when read with the UK National Annex to EN 1991-1-3, is very similar to BS 6399-3. The snow map in the UK National Annex is zoned with altitude adjustments, as opposed to that in BS 6399-3, which had isopleths, and it benefits from better analysis of the latest data from the metrological office [4].

1. INTRODUCTION.

The terminology adopted in the Eurocodes will be unfamiliar to the majority of designers and may prove an obstacle to the rapid uptake of the Eurocodes. Most of the definitions given in the Eurocodes derive from:

ISO 2394 (1998) General principles on reliability for structures
ISO 3898 (1997) Basis for design of structures – Notations – General symbols
ISO 8930 (1987) General principles on reliability for structures – List of equivalent terms

EN 1990 provides a basic list of terms and definitions which are applicable to all the other Eurocode parts, thus ensuring a common basis for the structural Eurocodes. This section has been provided to help to explain some of the key abbreviations, definitions and symbols used in the structural Eurocodes.

2 Abbreviations.

B Rules applicable only to buildings EHF Equivalent Horizontal Force EN European Standard EQU Associated with the loss of static equilibrium FAT Associated with fatigue failure of the structure or structural members GEO Associated with failure or excessive deformation of the ground I Informative N Normative NA National Annex NCCI Non-Conflicting Complementary Information P Principles STR Associated with internal failure or excessive deformation of the structure or structural members

2 Definitions.

Attention is drawn to the following key definitions, which may be different from current national practice:

Accidental action: An exceptional loading condition usually of high magnitude but short duration such as an explosion or impact.. Action: A load, or imposed deformation to which a structure is subjected (e. temperature effects or settlement).

Application rules: Clauses marked ‘P’ in the Eurocodes are principles, which must be followed. Clauses not marked ‘P’ are application rules which, when followed, satisfy the principles. Alternative design rules may be adopted. Application rules make up the bulk of the codes and give the values and formulae to be used in the design.

Characteristic: The typical (unfactored) value of a parameter to be used in design.

Co-existence: Eurocodes being in force in parallel with national codes. Combinations of actions: The combination of different sources of load acting simultaneously for the verification of structural reliability for a given limit state.

Conformity: Compliance with standards.

Design resistance: The capacity of the structure or element to resist the design load. Effects of actions: Internal moments and forces, bending moments, shear forces and deformations caused by actions.

Execution: All activities carried out for the physical completion of the work including procurement, the inspection and documentation thereof. The term covers work on site; it may also signify the fabrication of components off site and their subsequent erection on site.

Fatigue: A mode of failure in which a member ruptures after many applications of load. Fundamental combinations: Combinations of actions for the persistent or transient design situations.

Frequent: Likely to occur often, but for a short duration on each occasion.

Informative: For information, not a mandatory requirement – see normative. Load arrangement: Identification of the position, magnitude and direction of the loads (loading pattern).

Load case: Compatible loading arrangements considered simultaneously

Load combination: See ‘Combinations of actions’. National Annex: The document containing nationally determined parameters (NDPs). This is an essential supplement without which the Eurocode cannot be used.

NDPs: Nationally Determined Parameters. Values left open in a Eurocode for definition in the country concerned.

Non-Contradictory Complementary Information: Permitted additional information and guidance. Normative: Mandatory, having the force of a Standard.

Persistent: Likely to be present for most of the design life.

Principles: Clauses marked ‘P’ define structural performance that must be achieved.

2. ABBREVIATIONS, DEFINITIONS AND SYMBOLS.

Quasi-: Being partly or almost.

Quasi-permanent action: An action that applies for a large fraction of the design life.

Quasi-static: The static equivalent of a dynamic action.

Reference period: Any chosen period, but generally the design life.

Reliability: The mathematical probability of a structure fulfilling the design requirements.

Resistance: The capacity of a member or component to withstand actions without mechanical failure, e. bending resistance.

Transient: Likely to be present for a period much shorter than the design life but with a high probability of occurring.

Verify: Check the design output to make sure it complies.

2 Symbols (Greek letters).

The following Greek letters are used in EN 1990 and this document:

α (alpha) α A Reduction factor for area α n Reduction factor for number of storeys α cr Factor by which the design loads FEdwould have to be increased to cause global elastic instability at the load Fcr(i. α cr= F cr/ F Ed)

γ (gamma) Partial factor γ G Partial factor for permanent actions γ Q Partial factor for variable actions

ψ (psi) ψ 0 Factor for combination value of a variable action ψ 1 Factor for frequent value of a variable action ψ 2 Factor for quasi-permanent value of a variable action

ξ (xi) Reduction factor

Σ (sigma) Summation

Table 3: Design values of actions for strength (STR) using Equations 6 and 6

Persistent and Permanent actions Leading Accompanying transientdesign Unfavourable Favourable variable variable situations action actions

Eq. 6 1 G kj,sup 1 G kj,inf 1 ψ 0,i Q k,i

Eq. 6 ξ ×1 G kj,sup 1 G kj,inf 1 Q k,1 1 ψ 0,i Q k,i

The ξ factor that appears in Equation 6 of EN 1990 is a reduction factor for unfavourable permanent actions G. The UK National Annex sets the ξ factor equal to 0. When combined with γ Gin Equation 6 the effect is to reduce the overall factor from 1 to 1. In applying Equation 6 all variable actions are termed ‘accompanying’ (the largest of which is the main ‘accompanying action’), whereas in applying Equation 6 the most significant variable action is termed the ‘leading variable action’, and all others (i>1) are simply ‘accompanying’.

The combination factor ψ 0 that appears in each of Equations 6, 6 and 6 is one of three ψ factors ( ψ 0 , ψ 1 and ψ 2 ) used in EN 1990. The purpose of ψ 0 is to take account of the reduced probability of the simultaneous occurrence of two or more variable actions. ψ factors are discussed in Section 4.1 of EN 1990. Values for ψ factors for buildings in the UK are given in Table NA.A1 of BS EN 1990. In general, these factors are the same as those recommended in Table A1 of EN 1990, but with some exceptions. For example, ψ 0 is 0 for imposed loading on roofs and 0 for wind loading on buildings in EN 1990, whereas the UK National Annex gives values of 0 for imposed loading on roofs and 0 for wind loading. Selected values of ψ 0 from the UK National Annex are given in Table 3. Values of ψ 1 and ψ 2 from the UK National Annex are also provided in Table 3, but only feature in serviceability or accidental combinations.

Table 3: Values of ψ factors for buildings

Action ψ 0 ψ 1 ψ 2

Imposed loads in buildings, category (see EN 1991-1-1)

Category A: domestic, residential areas 0 0 0.

Category B: office areas 0 0 0.

Category C: congregation areas 0 0 0. Category D: shopping areas 0 0 0.

Category E: storage areas 1 0 0.

Category F: traffic area, vehicle weight ≤ 30 kN 0 0 0.

Category G: traffic area, 30 kN < vehicle weight ≤ 160 kN 0 0 0.

Category H: roofs 0 0 0

Snow loads on buildings (see EN 1991-1-3) - for sites located at altitude H > 1000 m above sea level 0 0 0. - for sites located at altitude H ≤ 1000 m above sea level 0 0 0

Wind loads on buildings (see EN 1991-1-4) 0 0 0

Temperature (non fire) in buildings (see EN 1991-1-5) 0 0 0

3 Serviceability limit states.

For serviceability limit states, guidance on combinations of actions is given in Clauses 6.5 and A1 of EN 1990. Three groups of combinations are identified: characteristic, frequent and quasi- permanent.

The characteristic combination is given by Equation 6 of EN 1990 and is normally used for irreversible limit states, such as permanent local damage or permanent unacceptable deformations.

Σ G k,j“+” P “+” Q k,1“+” Σ ψ 0,i Q k,i (6) j≥1 i>

The frequent combination is given by Equation 6 of EN 1 990 and is normally used for reversible limit states including excessive temporary (elastic) deformations or vibrations.

Σ G k,j“+” P “+” ψ 1,1 Q k,1“+” Σ ψ 2,i Q k,i (6) j≥1 i>

The quasi-permanent combination is given by Equation 6 of EN 1990 and is normally used for reversible limit states where long term effects are important (e. shrinkage, relaxation or creep). This is rarely applicable for steel structures.

Σ G k,j“+” P “+” Σ ψ 2,i Q k,i (6) j≥1 i>

The UK National Annex to EN 1993-1-1 (Clauses NA.2 and NA.2) states that vertical and horizontal deflections may be checked using the characteristic combination with variable loads only (i. permanent loads should not be included). Deflection limits are also provided, which are the same as those given in BS 5950. The basis for employing the characteristic combination is that excessive deflections may cause permanent local damage to connected parts or finishes (i. irreversible limit states), even though the steel members themselves will generally remain elastic.

In this section, Eurocode load combinations for multi-storey buildings are set out. General guidance for both simple and moment resisting frames is given in Section 4, since, in principle, load combinations are the same for both types of structure. However, differences in treatment often arise due to differences in sway stiffness, member interaction etc. and hence, specific guidance and examples for moment resisting and simple frames is provided in Sections 4 and 4, respectively. The following load categories are considered: Dead loads G k,imposed loads I k,snow loads S kand wind loads W k.

4 General.

4.1 Classification of frames An important classification of frames is in relation to their sway sensitivity. Adequate sway stiffness is important because it limits the lateral deflections of the frame and hence controls second order ( P- Δ) effects. Sway stiffness is assessed in EN 1993-1-1 in a similar way as it is in BS 5950, through the α crparameter (equivalent to λ cr in BS 5950), which represents the factor by which the design loading would have to be increased to cause overall elastic buckling of the frame in a global sway mode (Clause 5.2(3) of EN 1993-1- 1). A simplified means of determining α crfor regular frames is also given in Equation 5 of EN 1993-1-1. Regardless of the frame type (i. braced or moment resisting), if α cris greater than or equal to 10, the sway stiffness is deemed sufficiently large for second order effects to be ignored. Conversely, if α cris less than 10, second order effects may no longer be ignored. Second order effects are discussed further in Section 4.1.

4.1 Frame imperfections and equivalent horizontal forces (EHF) Frame imperfections may be incorporated directly into the structural analysis by defining an initial slant for the frame. However, the more general approach is to replace this geometric imperfection with a system of equivalent horizontal forces (EHF), referred to as notional horizontal loads in BS 5950. Whereas in BS 5950, equivalent horizontal forces were only required in the vertical load case, in the Eurocodes it is deemed that since frame imperfections are inherently present, they should be included in all ULS load combinations, since their purpose is to represent the initial imperfect geometry, from which deflections occur under the applied load. EHF are not required in SLS load combinations. The EHF should be determined separately for each load combination since they depend on the level of design vertical loads. For each storey, the EHF may be calculated as the design vertical load for that storey (not the cumulative vertical load) multiplied by 1/2 00 (i. 0%). Depending on the height of the structure and the number of columns contributing to the horizontal force on the bracing system, reductions to this basic value of 1/200 are possible, as detailed in Clause 5.3(3) of EN 1993-1-1. If horizontal loads ( H Ed) exceed 15% of vertical loads ( V Ed) these sway imperfections may be disregarded, and EHF ignored – this would more often apply to low rise buildings.

4.1 Second order ( P-Δ ) effects Second order effects relate to the increase in member forces and moments that occur as a result of deformation of the structure under load. As outlined in Section 4.1, second order ( P- Δ) effects need not be considered provided the frame is sufficiently

stiff (i. sway deformation under the design loading is relatively small) – this is deemed to be the case for elastic analysis when α cr ≥ 10, and similarly, according to the UK National Annex, for plastic analysis of clad frames when the additional stiffening effect of the cladding has been neglected. In cases where α cris less than 10, the designer is presented with a number of options. These include enhancement of the stability system such that α cris raised above 10 and hence second order effects may be ignored, making allowance for second order effects by approximate means (amplified sway method or effective length method, both of which were allowed in BS 5950), or making allowance for second order effects by performing a second order structural analysis enabling and accounting for deformation of the structure under load. It should be noted that if α cris less than 3, then an accurate second order analysis must be performed (Clause 5.2(5) of EN 1993-1- 1). The aforementioned is summarised in Table 4.

Table 4: Summary of analysis methods and treatment of second order effects

Limits on α cr Analysis method Result α cr≥ 10 First order analysis Second order effects ignored 10 > α cr≥ 3 First order analysis plus Second order effects amplified sway method or allowed for by effective length method approximate means α cr< 3 Second order analysis Second order effects allowed for more accurately

The most common approximate treatment of second order effects in multi-storey buildings, which may be applied provided that α cr ≥3, is the so called ‘amplified sway method’. In this method, account for second order effects is made by amplifying all lateral loading on the structure (typically wind loads and EHF) by a factor, referred to in the UK National Annex to EN 1993-1-1 as k r, which is related to the sway stiffness of the structure through Equation D4 (Equation 5 of EN 1993-1-1).

k r= 1 (D4) 1-1/ α cr

4.1 Reduction factors for number of storeys ( α n) and floor area ( α A) As the number of storeys in a building increase, the likelihood that all floors will be loaded to the full design level decreases. Similarly, large floor areas will seldom be subjected to the full design loading uniformly. To reflect this, reduction factors for imposed loads may be applied for the design of floors, beams and roofs and for the design of columns and walls. For the design of individual floors, beams and roofs, the area reduction factor α Amay be applied. For the design of columns and walls, the reduction factor α nfor the number of storeys may be applied. The reduction factor α nrelates to the number of floors supported by the column section under consideration, and may be applied to the total imposed load being carried. If, for a given column or wall, α A< α n, then α Amay be used in place of α n, but α Aand α nmay not be used together (Clause NA.2).

4. MULTI-STOREY BUILDINGS.

4 Moment resisting frames ( continuous construction).

(continuous construction)

Moment resisting frames are statically indeterminate. There is interaction between the members and so load combinations need to be considered for the full structure. For simple braced frames, the individual members can essentially be designed in isolation enabling more straight-forward load combinations, as described in Section 4. Unbraced (moment resisting) frames are also generally less stiff laterally than braced frames, and are therefore more likely to require consideration of second order effects.

4.2 ULS load combinations based on Equation 6 with α cr≥ 10 For frames with α cr≥ 10, second order effects need not be considered. The basic gravity load combination (i. dead load + imposed load) arising from Equation 6 of EN 1990 is given by Equation D4:

Gravity only 1 G k“+” 1 l k (floors) “+” 1( I k,roofor S k) (roof) “+” (D4)

Equation D4 applies to the full building – for the floors, the imposed floor loading I kshould be adopted, whilst for the roof, the higher of the imposed roof load I k,roofand the snow load S kshould be used. Since the variable gravity load on the roof will be either the imposed load or the snow load (i. snow and imposed roof load are not to be considered simultaneously – see Clause 3.3(1) of EN 1991-1-1), both are considered to be the leading variable action, attracting a load factor of 1.

Considering wind loading, for cases of wind uplift W k,up, gravity loads are favourable since they oppose the uplift forces. In such cases, the dead load is assigned a load factor of 1, whilst the imposed load (or snow load) has a load factor of zero. This results in Equation D4.

1 G k“+” 1 W k,up“+” EHF Wind uplift (D4)

Considering dead, imposed and wind loads acting together, and assuming all loads to be always unfavourable (i. causing an increase in member forces or moments), two further load combinations, given by Equations D4 and D4, arise from Equation 6 of EN 1990. In Equation D4, imposed load is assumed to be the leading variable action and hence attracts a load factor of 1, whilst the wind load W kis reduced by a combination factor ψ 0 of 0 (to give a load factor = 0 x 1 = 0). Note that, at the roof level, the imposed load should not be considered in combination with either the snow load or the wind load (see Clause 3.3(1) of EN 1991-1-1). Hence, in Equation D4, the imposed floor load I kis applied to the floors and the snow load S kis applied to the roof, with both considered to be the leading variable action, with a load factor of 1, at their location.

Gravity leading + Wind 1 G k“+” 1 I k (floors) “+” 1 S k (roof) “+” 0 W k“+” EHF(D4)

In Equation D4, the wind load is now considered as the leading variable action with a load factor of 1, thus the imposed load is reduced by a combination factor ψ 0 of 0 (applicable in all cases except for storage areas), to give a load factor = 0 x 1 = 1. Again, since this load combination features wind loading, the snow load, which has a value of ψ 0 = 0 (at altitudes of less than 1000m), should be applied to the roof to give a load factor = 0 x 1 = 0.

Gravity + Wind leading 1 G k“+” 1 W k“+” 1 I k (floors) “+” 0 S k (roof) “+” EHF(D4)

4.2 ULS load combinations based on Equation 6 with α cr< 10 For frames with αcr< 10, second order effects must be considered. This may be avoided by appropriate reconfiguration of the bracing system in order to increase the sway stiffness of the structure and hence ensure α cr≥ 10, though simply increasing the cross-sectional area of the bracing to achieve this will generally prove to be uneconomical. Otherwise, account must be made of second order effects. For α cr< 3, an accurate second order analysis is required, while for regular frames with α cr≥ 3 approximate methods to allow for second order effects may be employed, the most common of which is the amplified sway method. In this case, load combinations will be the same as those defined in Section 4.2, except that all horizontal loads ( W k+ EHF) and other possible sway effects (e. arising from asymmetric loading) will be multiplied by k r(Equation D4). Note that k ris derived from α cr, which is in turn dependent on the loading F Edon the structure, so, as for EHF, k rshould be determined separately for each load combination.

4.2 ULS load combinations based on Equation 6 and 6 with α cr ≥ 10 Considering load combinations from Equation 6 and 6 of EN 1990, as explained in Section 3, unless the dead load is substantially greater than the imposed load, the governing load combinations will be derived from Equation 6, and Equation 6 will not normally need to be considered. The only difference between Equation 6 and Equation 6 is that Equation 6 will have a lower dead load factor of 1 due to the introduction of the ξ factor with a UK National Annex value of 0 (see Section 3). Noting that ξ is a reduction factor on unfavourable dead loads, and hence will not affect the wind uplift combination where the dead load is favourable, the load combinations given by Equations D4 to D4 (derived from Equation 6) now (by applying Equation 6) become:

Gravity only 1 G k“+” 1 I k (floors) “+” 1( I k,roofor S k) (roof) “+” EHF (D4)

Wind uplift 1 G k“+” 1 W k,up“+” EHF (D4)

Gravity leading + Wind 1 G k“+” 1 I k (floors) “+” 1 S k (roof) “+” 0 W k“+” EHF (D4)

Gravity + Wind leading 1 G k“+” 1 W k“+” 1 I k (floors) “+” 0 S k (roof) “+” EHF(D4)

Equations D4 to D4 represent the four basic load combinations for multi-storey frames. For economy, it is recommended that these load combinations (Equations D4 to D4 all emerging from Equation 6) be used in preference to those arising from Equation 6 (Equation D4 to D4). 14

4.2 ULS load combinations based on Equations 6 and 6 with α cr< 10 As described in Section 4.2, when α cr< 10, second order effects must be considered. If the amplified sway method is employed, load combinations will be the same as those given in Equations D4 to D4, except that all horizontal loads (wind and equivalent horizontal forces) and other sway effects are multiplied by the factor k r, which, as noted in Section 4.2 is load combination dependent.

4.2 SLS load combinations As outlined in Section 3, the UK National Annex to EN 1993-1- states that vertical and horizontal deflections may be checked using the characteristic combination with variable loads only (i. permanent loads should not be included). The characteristic combination is defined by Equation 6 of EN 1990, where the leading variable action is unfactored (i. taken as its characteristic value) and all accompanying variable actions are reduced by the combination factor ψ 0.

Assuming all loads to be unfavourable, the resulting SLS combinations are given by Equations D4 (where imposed load or snow load on the roof is taken as the leading variable action) and D4 (where wind load is taken as the leading variable action).

1 I k(floors) “+” 1 S k(roof) “+” 0 W k (D4)

1 W k“+” 0 I k(floors) “+” 0 S k(roof) (D4)

For cases where the influence of horizontal loading on vertical deflections is deemed insignificant, or for cases where wind load is favourable (e. suction on a roof may reduce deflections), Equation D4 reduces simply to Equation D4 (i. checking vertical deflections under unfactored imposed or snow loading only).

1 I k(floors) “+” 1( I k roofor S k) (roof) (D4)

For cases where the influence of vertical loading on horizontal deflections is deemed insignificant, or for cases where vertical loading is favourable, Equation D4 reduces to Equation D4 (i. checking horizontal deflections under unfactored wind loading only).

1 W k (D4)

Deflection limits are also provided in the UK National Annex to EN 1993-1-1 in Clauses NA.2 and NA.2. The deflection limits of relevance to multi-storey buildings, which are the same as those given in BS 5950, are presented in Tables 4 and 4.

Table 4: Vertical deflection limits

Vertical deflection Limit Cantilevers Length/

Beam carrying plaster or other brittle finish Span/36 0

Other beams (except purlins and sheeting rails) Span/

Table 4: Horizontal deflection limits

Horizontal deflection Limit

In each storey of a building with more Height of than one storey that storey/

4 Braced frames (simple construction).

In simple braced frames, load combinations and design calculations can be simplified by separating the treatment of different groups of members. Four groups of members, namely roof beams, floor beams, columns, and columns forming part of the bracing system, are considered under the following two sub- sections, which address load combinations according to Equation 6 and Equations 6 and 6, respectively. Note that in simple braced frames, equivalent horizontal forces (EHF) and second order effects need only be considered for the bracing members and the columns that form part of the bracing system.

4.3 ULS load combinations based on Equation 6 0

ROOF BEAMS For roof beams, four load combinations should be considered. The first considers gravity loads only, in which the variable action is taken as the higher of the imposed roof load and the snow load.

1 G k“+” 1( I k,roofor S k) (roof) Gravity only (D4)

The wind uplift combination is given by: 1 G k“+” 1 W k,up Wind uplift (D4)

The final two combinations consider dead load, snow load and wind load, with snow leading (Equation D4) and wind leading (Equation D4).

1 G k“+” 1 S k(roof) “+” 0 W kGravity leading + Wind (D4)

1 G k“+” 1 W k“+” 0 S k(roof)Gravity + Wind leading (D4)

FLOOR BEAMS For floor beams, only the gravity load combination needs to be applied:

1 G k“+” 1 I k Gravity only (D4)

COLUMNS For columns, the gravity load only combination, with the higher of the imposed roof load and the snow load applied at roof level, is given by Equation D4:

1 G k“+” 1 I k(floors) “+” 1( I k,roofor S k) (roof)Gravity only (D4)

Where the wind load also has a downward vertical component at the roof level, the following two combinations should also be assessed:

Gravity leading + Wind 1 G k“+” 1 I k(floors) “+” 1 S k(roof) “+” 0 W k (D4)

Gravity + Wind leading 1 G k“+” 1 W k“+” 1 I k(floors) “+” 0 S k(roof) (D4)

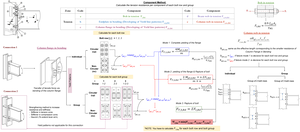
Figure 4: Cross-section of building, bracing configuration and loading

The design (factored) loads on the roof beams, floor beams and columns in the internal frames, and bracing and columns in the end frames that contain diagonal cross-bracing, are calculated, based on the load combinations given by Equations D4 to D4, and presented in Tables 4 to 4. The maximum design uniformly distributed loads (UDLs) and forces are marked in bold, indicating the critical load combinations.

Table 4: Design UDLs (kN/m) on roof beams of internal frames

Table 4: Design UDLs (kN/m) on floor beams of internal frames that do not contain bracing

G k,roof; I k,roof; S k; W k; W k up

Outer column

G k; I k

Inner column

Bracing (in end frames)

5m 5m 5m

W k W k Equation

ULS Design UDL q Ed

Vertical W k,up = -0. kN/m 2

Vertical W k= 0. kN/m 2

S k= 0. kN/m 2

I k,roof = 1. kN/m 2

G k,roof = 3. kN/m 2

Load Combination

Characteristic 21/m kN/m9 kN/m3 kN/m0 -0/m -

D4 Gravity 26/m kN/m13 - - - 39/m

D4 Wind Uplift 21/m - - - -0/m 20/m

D4 26/m - kN/m4 kN/m0 - 31/m

Gravity leading + Wind

D4 26/m - kN/m2 kN/m0 - 29/m

Gravity leading + Wind

Equation

ULS Design UDL q Ed

I k = 5. kN/m 2

G k= 3. kN/m 2

Load Combination

Characteristic 21/m 30/m -

D4 Gravity 26/m 45/m 71/m

Equation

Design axial force in inner columns N Ed

Vertical W k= 0 kN/m 2

I k = 5 kN/m 2

S k= 0. kN/m 2

I k,roof = 1 kN/m 2

G k,roof= 3. Load Combination kN/m 2

Characteristic 21 kN/m 9 kN/m 3 kN/m 30 kN/m 0 kN/m -

D4 Gravity 26 kN/m 13 kN/m - 45 kN/m - 1267 kN

D4 Gravity leading + Wind 26 kN/m - 4 kN/m 45 kN/m 0 kN/m 1224 kN

D4 1013 kN

Design axial force in outer columns N Ed

633 kN

612 kN

2 kN/m 31 kN/m 0 kN/m 506 kN

G k= 3. kN/m 2

21 kN/m

26 kN/m

Gravity + Wind leading 26 kN/m - 26 kN/m

Equation

Force due to lateral W k= 0. kN/m 2 (roof level)

Vertical W k= 0. kN/m 2

I k = 5. kN/m 2

S k= 0. kN/m 2

I k,roof = 1. kN/m 2

G k,roof= Load Combination3 kN/m 2

Characteristic 10/m kN/m4 kN/m1 15/m kN/m0 24 kN

D4 Gravity + EHF 13/m kN/m6 - 22/m - -

D4 Gravity leading +Wind + EHF 13/m - kN/m2 22/m 0/m 18 kN

D4 36 kN

Force due to lateral W k= 0. kN/m 2 (floor level)

36 kN

72 kN

EHF (roof level)

4 kN

3 kN

EHF (floor level)

7 kN

6 kN

Design axial tensile force in bracing N Ed

34 kN

190 kN

341 kN

Design axial force in inner braced columns N Ed

653 kN

723 kN

kN/m1 15/m kN/m0 706 kN

G k= 3. kN/m 2

kN/m
kN/m
kN/m

kN/m

kN/m

Gravity + Wind + EHF

Table 4: Design UDLs on frame (kN/m) and design axial forces (kN) in bottom storey columns of internal frames that do not contain bracing

Table 4: Design UDLs on frame and design axial forces (kN) in bottom storey bracing and columns of end frames that contain diagonal cross-bracing

5 General.

Although industrial buildings can be designed to support mezzanine floors and cranes, they are primarily loaded by their self weight, service loads, imposed loads or snow loads and wind loads. Service loads tend to be ‘project specific’ but a nominal value of around 0 kN/m 2 should always be considered in structural design to allow for loads from nominal lighting. This value will increase if more substantial services such as sprinkler systems or air-conditioning are incorporated. The self weights of false ceilings over intermediate floors are often also treated as service loads. Snow loads and wind loads are site specific and are influenced by the geometry of the structure and its orientation. Snow loads are determined by reference to EN 1991-1-3 and its UK National Annex. Wind loads are determined by reference to EN 1991-1-4 and its UK National Annex, but designers might also like to refer to Reference [5] for further guidance.

Clause 3.3 (1) of EN 1991-1-1 states that on roofs, imposed loads and snow loads or wind loads should not be applied together simultaneously. This means (1) that snow load and imposed load should not appear together in any given load combination, and (2) that imposed load and wind load should not appear together in any given load combination. The basis for this clause is that it would be unreasonable to consider that maintenance would be undertaken in severe weather conditions.

The concept of ψ factors was introduced in Section 3 and Table 5. presents the ψ factors that are relevant to portal frame design. In Table 5, G kc= permanent crane action and G kc+ Q kc= total crane action (from Clause A.2 of EN 1991-3 Annex A).

Table 5: ψfactors relevant to portal frame structures

ψ 0 ψ 1 ψ 2 Imposed loads on roofs 0 0 0. Snow loads at altitude less than or equal to 1000 m 0 0 0. Wind loads 0 0 0. Crane loads 1 0 G kc/( G kc+ Q kc)

5.1 EN 1991-1-3: 2003 - Snow loading In Section 2 of EN 1991-1-3, ‘Classification of actions’, snow loads are classified as variable fixed actions unless otherwise specified in the code. In this section it also states that exceptional snow loads and exceptional snow drifts may be treated as accidental actions, depending on geographical locations. The UK National Annex confirms this in Clauses NA.2 and NA.2 and also states that Annex B should be used to determine the drifted snow load case. This approach is consistent with current UK practice for designers using BS 6399-3 and BRE Digest 439 [8] to determine uniform snow loads and the loads caused by drifted snow.

5.1 EN 1991-1-4: 2003 - Wind loading Wind actions are defined as variable fixed actions. The process for determining wind pressures is based on a 10-minute mean wind velocity and a new map has been provided in the UK National Annex. Designers who have been working with BS 6399-2 will find

the approach for determining wind pressures very similar although some terminology has changed. The publication “Designers’ Guide to EN 1991-1-4 Eurocode 1: Actions on structures, general actions part 1-4. Wind actions” [5] is very important in explaining the background and limitations of the new European Standard.

Although wind pressures vary depending on site location, altitude, orientation etc, the pressure and force coefficients depend only on the external shape of the structure. By looking at the overall pressure coefficients, irrespective of the actual site wind pressures, it is possible to determine the critical load cases. For a portal frame with a roof pitch of 5°, Figure 5 shows the external pressure coefficients, c pc, while the overall pressure coefficients (internal and external) are presented in Figure 5.

External pressure coefficients for the walls have been extracted from Table 7 of EN 1991-1-4 assuming an h/d ratio ≤ 0, while those for the roof have been extracted from Tables 7 and 7. Once the basic external coefficients have been established, to comply with the requirements of Clauses 5 and 7.2 of EN 1991-1-4, two additional factors are applied:

The structural factor c s c d– for the majority of portal frames the height will be less than 15 m and the value of c s c dis taken as
For buildings with h/d ≤1, which covers most portal frames, the external horizontal wind forces on the windward and leeward faces (i. under transverse wind loading) are multiplied by 0.

Internal pressure coefficients c pi for buildings with uniformly distributed openings are determined from Figure 7 of EN 1991- 1-4. Values of the internal pressure coefficients depend on the h/d ratio of the building and the parameter μ , which is the ratio between the sum of the areas of openings where the external pressure coefficient is zero or negative and the sum of the areas of all openings. For longitudinal wind load cases, the external pressure coefficients will be predominantly negative, hence the value of μ will be close to unity and, from Figure 7 of EN 1991- 1-4, assuming h/d ≤ 0, c piwill be approximately -0. For transverse wind load cases, μ will be lower and hence higher values of c piwill be found from Figure 7. Note 2 of clause 7.2(6) of EN 1991-1-4 states that c pivalues may be estimated as the more onerous of 0 and -0. This, however, may prove to be overly conservative, and it is recommended that designers make use of Figure 7 to determine the specific values of c pifor their building. For the example presented herein, 0.0/-0 is used for the longitudinal wind load case, with 0 clearly being the more critical, while 0.2/-0 is used for the transverse wind load cases.

5. INDUSTRIAL BUILDINGS.

Multiple Choice

Multiple Choice

Was this document helpful?