- Information
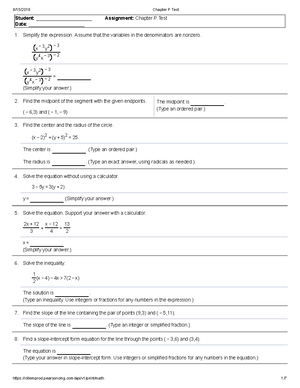
- AI Chat
Was this document helpful?
Pre calc - the imaginary unit i notes (page 1)
Subject: Pre-Calculus
397 Documents
Students shared 397 documents in this course
Level:
Standard
Was this document helpful?

The imaginary unit i notes
Intro to the imaginary numbers:
-Some quadratic equations do not have any real number solutions.
for example:
you will never be able to find a real number solution to the equation . The reason behind this is because it is impossible to square a real number and get a negative
number. The solution to the equation does exist in a new number system called the complex number system.
The imaginary unit:
-The backbone of this new system in the imaginary unit, or the number i.
The problems down below is true of the number i
The second property shows that the number i is a solution to the equation up above. This question was previously unsolvable which is now solvable with the addition
of the imaginary unit.
Pure imaginary numbers:
-The number i is not alone. We can create many more pure imaginary numbers.
for example:
-These are all examples of pure imaginary numbers. Numbers of the form bi, where b is a nonzero real number.
-Taking the squares of these numbers helps people understand how they relate to the real numbers.
Example:
If you square the number 3i the properties of integer exponents remain the same, so we can square 3i just as we would think.
-The fact that (3i)over 2 = 9 means that 3i is a square root of -9
using the fact that i over 2 = -1,
we can simplify this further
Check your understanding:
Simplifying pure imaginary numbers
-The table below shows examples of pure imaginary numbers in both unsimplified and in simplified form.
The square root of -9 is an imaginary
number. The square root of 9 is 3, so
the square root of negative 9 is 3
imaginary units or 3i
1
-
23
-
23
x
=
=
-
1
i
=
F1
j
=
-
1
30,255,
and
-
120
(33)2
=
3232
=
p22
=
p;2
=
P(
-
1)
=
-
q
What
is
(4)??
(4i)2
=
y2;z
=
(biz
=
16(
-
1)
=
-
lb
which
of
the
following
is
a
square
root
of
-16?
4)
The
fact
that
19:):-16
means
that
4
is
a
square
root
of
-16.
The
number
-
4
is
a
square
root
of
Positive
16
Since
1-4)2
=
16.)
unsimplified
Simplified
form
5
·
35
FS
:5
-
VY4
-
125
78
=
35