- Information
- AI Chat
Normal Distributions Test Review KEY
Pre-Calculus
Standard
Recommended for you
Preview text
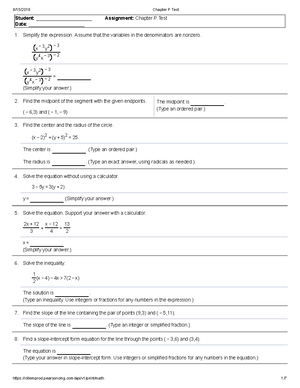
M436 Statistics Name: _________________________________ Normal Distributions Review A set of 3000 golf scores has a normal distribution. The mean of the data is a score of 89, and the standard deviation is 5. (Use this information to answer questions 1 – 11)
- Sketch the curve.
- Find the interval of scores that are within one standard deviation of the mean.
- Use the Empirical Rule to estimate the percentage of golfers that scored between a 74 and a 94.
- What is the z score for 96?
- What percent of the data is less than 87?
Key
Fifita
84,
68 13 2.
83.
Z X M O Z 96. 89 s 1. normalcdf lower 10000 upper
87 34.
μ 89 0 5
- What percent of the data is between 87 and 96?
- What percent of the data is between 10 and 180?
- What is the golf score of a person who has a percentile score of 70?
- What percent of the data is greater than 95?
- What is the interval about the mean that includes 82% of the data?
- What percentage of data is between -0 and 1 standard deviations of the mean? normalcdf lower 87 upper 96 57. μ 89 0 normalcdf lower 10 upper 180 100 μ 89 0 5 invnorm area 70 u 89 r s
- normalcdf lower 95 upper 10000
- μ 89 0 5
####### μg
invnorm area 09 arg 9 mg
I
PEE Eg
Norm 182 95. normalcalf lower 2 upper 1 48.
SAT and ACT scores are normally distributed. The mean SAT score is 1000, with a standard deviation of 100. The mean ACT score is 18, with a standard deviation of 4. 16. What is the z-score of a student who scores a 24 on the ACT? 17. If a student scores a 24 on the ACT, what would you predict that same student to score on the SAT? Assume golf scores are normally distributed. The mean golf score at Course A is 96, with a standard deviation of 8. The mean golf score at Course B is 88, with a standard deviation of 4. 18. If a golfer shoots a 90 at Course A, what would you predict that same golfer to shoot at Course B? SAT ACT M 1000 M 18 2 24 418 1. 0 100 4 100 5 46800. 1150 158
7
A B
8
Ez score 2 90 96 75 from A to predict B 8 4 75 X
4881
85 3 4 88 88 88
Normal Distributions Test Review KEY
Subject: Pre-Calculus
Standard
