- Information
- AI Chat
Was this document helpful?
Lab report - coeffcient of friction
Course: General Physics I-Trig Based (PHY 201)
34 Documents
Students shared 34 documents in this course
University: Jefferson Community College (New York)
Was this document helpful?

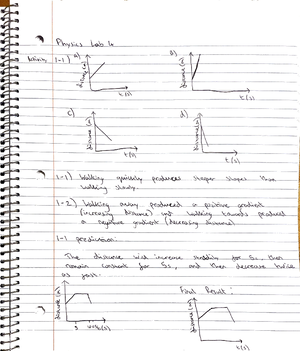
Coefficient of friction
Objective: To investigate the nature of the frictional forces between a smooth wooden block
and a flat wooden board, in the horizontal and inclined plane.
Factors:
1. ms Coefficient of static friction
2. mk Coefficient of kinetic friction
3. N Normal force of the block
4. fs Frictional force (static)
5. fk Frictional force (kinetic)
6. m Mass
7. g Gravity
8. Angle of the inclined plane
9. T Tension of the string
Theory:
Friction is a resisting force that occurs when one object slides or attempts to slide over
another. This force always acts at a tangent to the two surfaces, and in the opposite
direction of the motion, or attempted motion. The friction depends upon the roughness of
each surface, and the normal force, which is the force that the objects exert on each other.
The normal force is always perpendicular to the surface.
The frictional force is directly proportional to the normal force, so the normal force (N)
multiplied by a constant (µ) is equal to the frictional force. Note that the value for µ is
dependent on the objects in contact, and if there is motion or not.
There are two types of friction, static friction (fs) and kinetic friction (fk), therefore we get
the equations:
1) fs = µsN
2) fk = µkN
We put µs and µk because the value of µs is larger than µk, meaning the static frictional
force is larger than the kinetic frictional force.
If we consider an inclined plane with an angle s, where s is the angle that the block begins
to move, then we can find the normal force using:
3) N = mgcoss
And we can find fs using:
4) fs = mgsins
Combining these equations, we get: