- Information
- AI Chat
Was this document helpful?
Math 11-Worksheet 04 - worksheet
Course: Calculus I (MATH 011)
81 Documents
Students shared 81 documents in this course
University: University of California, Merced
Was this document helpful?

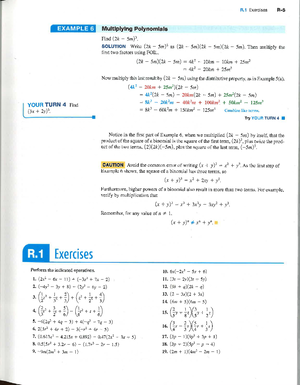
Math 11 Worksheet 4 - Sections 3.1 and 3.2a Spring 2023
1 Section 3.1
1. At time tin seconds, a particle’s distance s(t), in micrometers (µm), from a point is given by
s(t) = 3
4t2−1
What is the average velocity of the particle from t= 2 to t= 4?
2. Consider the graph below of the position of a model rocket, h(t). Here his in meters and tis measured
in seconds.
(a) Estimate the average velocity of the rocket in the first 3 seconds of flight.
(b) What is the average velocity of the rocket in the last 3 seconds of flight?
(c) Estimate the average velocity of the rocket between 1 and 3 seconds.
(d) Estimate the average velocity of the rocket in the last 5 seconds of flight.
(e) In general, under what conditions is the average velocity for the rocket zero?
3. For each of the following situations, sketch a graph of the distance the car has traveled as a function
of time.
(a) A car is driven at a constant speed.
(b) A car is driven at an increasing speed.
(c) A car starts at a high speed, and its speed then decreases slowly.
4. Write out and simplify the difference quotient f(x+h)−f(x)
hfor each of the following functions.
(a) f(x) = 2x2+ 3 (b) g(x) = 4
x
1