- Information
- AI Chat
Gyroscopes - Lecture notes 1
Process Control Systems 3B
Durban University of Technology
Preview text
GYROSCOPES
Suppose a circular disk having its axis mounted in gimbals (see Fig. 1) is given a spin of angular velocityo. If the outer gimbal is turned through an angle, the spin axis of the disk will tend to point in the same direction as previously (see Fig. 1). This assumes of course that friction at the gimbal bearings is negligible.
In general the direction of the spin axis remains fixed even when the outer gimbal, which is attached to some object, moves freely in space. Because of this property the mechanism, which is called a gyroscope, finds many applications in cases where maintaining direction (or following some specified course) is important, as for example in navigation and guidance or control of ships, aeroplanes, submarines, missiles, satellites or other moving vehicles.
A gyroscope is another example of a symmetric spinning rigid body with one point on the symmetry axis (usually the centre of mass) taken as fixed.
I
er gimbal w
Figure 1 Outer
Figure 1.
1 GYROSCOPIC COUPLE
Ref. H A page 227, paragraph 10.
Let a disc, of polar moment of inertia I, rotate about its axis OX, Fig. 1, at o rad/s and let this axis be rotated (or precessed) in the horizontal plane XOZ about the axis OY at Q rad/s.
-3_ I
a
x
Spin
If this change takes place in time dt, Rate of change in angular momentum dt
but — Q (Angular velocity about axis OY) dt
therefore
Gyroscopic couple, C = 10 Q (1)
Applying the corkscrew rule to the vector ab, ( Fig. 1), the couple required to cause precession is directed along the OZ axis, clockwise looking in the direction ZO.
The reaction to this couple, i. the couple exerted by the shaft OX on its bearings, is opposite in direction to the applied couple.
It will be seen from Fig. 10 that the axes of spin, precession and couple are mutually perpendicular
The disc also has an angular momentum IdQ about the axis OY, Id being the moment of inertia about the diameter, but this momentum is not being depressed, (no change in direction of axis O Y), hence no gyroscopic couple is induced.
-5_ 1
1 EFFECT OF GYROSCOPIC COUPLE
Ref. H A page 228, paragraph 10.
Gyroscopic motion arises when ever the axis of a rotating body is caused to change direction. Examples may be found in the rotor of a turbine which is pitching in a ship, in the wheels of a vehicle turning round a bend and in gyroscopic instruments.
In every case, the couple which must be applied to the rotating body to cause it to precess is determined from a vector diagram, such as in Fig. 1, using the corkscrew rule, and the reactzon whzch the body applies to its bearings then obtamed.
In the case ofa ship's turbine rotor, the reaction couple on the shp causes it to swmg sideways.
In a vehicle, the reaction couple caused by the precession of the wheels tends to overturn the vehicle m the same way as the centrifugalforce.
I
1 ALTERNATIVE DERIVATION OF GYROSCOPIC COUPLE
Figure 1 shows three views of the disc, rotating about axes OX and OY with angular velocities o and Q rad/s respectively.
Hence Acceleration of P, perpendicular to the plane of the disc, = 2(o y) Q, (Fig. 1(c).
Note: 2(oy) Q is the coriolis acceleration, derived in mechanics of machines iii , or see derivation in your text book, paragraph 2 page 55.
Similarly, particles below the ZZ axis are moving to the left and therefore have an acceleration 2(o y)Q acting in the opposite direction; the accelerating forces on particles above and below ZZ then produce a couple about ZZ
Force to accelerate particle = mass x acceleration dm x 2(o y)Q
Therefore:
Moment of force about ZZ — Force x perpendicular distance
Therefore
Moment of force about ZZ — dm x 2(o y)Q x y = dm x 20 Q
Therefore
Total moment about ZZ, C = 20 Q f dm
i. C- 20 Q
But
21zz = I , the polar moment of inertia of the disc, Therefore
C = 10 Q
Note: The projection of p on yy axis moves with s.h. but the force necessary to produce this motion has no moment about zz axis.
The above result is only applicable to a bodyfor which the value ofIzz remains constant as it revolves.
1 GYROSCOPIC COUPLE ON A THIN ROD ROTATING IN A VERTICAL PLANE ABOUT A HORIZONTAL AXIS THROUGH ITS CENTRE
Consider a thin rod rotating in the vertical plane about a horizontal axis through its centre,
The accelerating force on a particle of mass dm at P has a moment about the axis AA of magnitude dm x20 y Q x r but Y = r sino
Now If
e = 0 0 and 180 0 Coz = 0
and if
e = 90 0 and 270 0
Coz = 210 Q (maximum value)
Couple about O Y, coy = (210Q sin 0) cose
If
9 = 0 0 , 90 0 , 180 0 and 270 0 Coy = 0
and if
0 = 45 0 , 135 0 , 225 0 and 270 0 Coy = 10 Q (maximum value)
The thin rod is the idealised case of a two-bladed propeller.
I
1 WORKED EXAMPLES
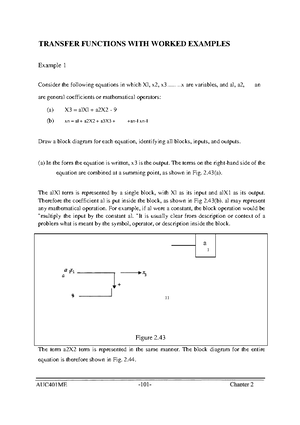
Example 1
Ref. H A Page 231, example 1 See question in text book
Solution
Maxzmum proscopzc torque caused. Moment of inertia of driving axle: I = 350 kg 2
Wheel diameter: D 1,85 m
Therefore
Wheel radius: r 0,925 m
Distance between planes of the wheels: I 1,5 m 5 Speed of train: v = 100 km/h= 100 x = 27,778 m/s 18
Periodic time:
From Fig. 10 (text book)
Rise and fall of 12 mm with S.H gives 2 x amplitude = 12
Therefore Amplitude = 6 mm = 6 x 10-3 m
Therefore
Q = 62,832 x 0,004 = 0,2135 rad/s
Maximum gyroscopic torque, C = I (D Q from equation (l. 1)
Therefore 350 x 30,03 x 0,2513 = 2 641 8 N
View ofwheels and axle with gyroscopic couple when the wheel is falling. Consider Fig. 1.
a
Figure 1.
When the right-hand wheel falls, the momentum vector oa moves to ob. Thus the couple for precession is clockwise looking upward (corkscrew rule) and the reaction couple exerted by
the wheels is equal and opposite, i. anticlockwise looking upward.
The locomotive thus tends to swing to the right.
couple
t Pp
i. 27t
Therefore
p = 0,5236 rad/s
Angular amplitude of motion, 7,5 0 x = 0, 1309 rad
Maximum velocity of precession, Q = i. Q = 05236 x 0,
Therefore Q = 0,0685539 rasd/s
Angular velocity of turbine rotor, 27tN 2TtX2 400 -251,33 rad/s 60 60
Moment of inertia of rotor, 1 =mk2 10,8x10 3 kg 2
Maximum gyroscopic couple, C = IoQ from equation (1)
i. c = 10,8 x 10 3 x 251,33 x 0,
Therefore C = 186 04 kN
Figure 1(b) shows a side view of the ship. When the bow rises, the momentum vector oa moves to ab. Thus the couple required for precession is clockwise looking upward and the reaction of
Example 3
Ref. H A page 233, example 3. See question in text book.
Solution
Limiting speed of the vehzcle round the curve for all four wheels to maintain contact With the road surface, ifthzs is not cambered.
Track mean radius
Moment of inertia of each wheel
Effective diameter of wheels
Therefore
Wheel radius Moment of inertia, rotating parts of engine Gear ratio Mass of vehicle Position of centre of gravity above ground level Width of the track of vehicle
Draw a sketch of the specified automobile
Moment of inertia of wheels
Iw = 4 x = 6,4 kg 2
Moment of inertia of engine — 0,85 kg 2 R = 100 m kg D 600 mm
r = 300 mm = 0 , 3 m le = 0, kg 2
M = 1 400 kg h = 450 mm = 0,45 m
Therefore
Angular velocity of wheels: rad / s But
Gyroscopes - Lecture notes 1
Course: Process Control Systems 3B
University: Durban University of Technology

- Discover more from: