- Information
- AI Chat
Multi-input Systems - Lecture notes 11
Process Control Systems 3B
Durban University of Technology
Preview text
Warning: TT: undefined function: 32
Multi-input Systems
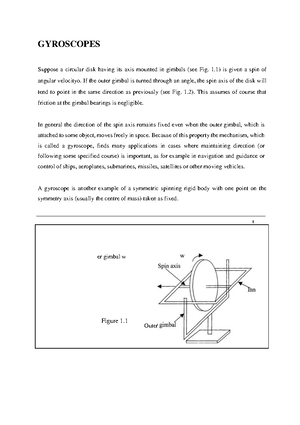
Often it is necessary to analyze and study a system in which more than one input is simultaneously applied at different points of the system. When multiple inputs are present in a linear system, the superposition theorem may be used. That is, each input is considered alone, setting others equal to zero. The final solution is then obtained by adding the individual answers together.
Consider the multi-input system of Fig. 2 in which R is the input and U represents the total noise and unwanted signals. Assuming that the system is linear, each input will be considered separately.
Figure 2.
c
Thus, let U = 0, and CR be the output due to R only. then system reduces to: [Fig. 2(a)].
Figure 2)
Then, replace the cascaded elements with one element Fig. 2(b).
Figure 2)
Consider loop as an open loop: Fig. 2(b).
i. Rx GIG2 - x H x i.
i. R x GIG2 = (1+ GIG2 x H) therefore GIG 1 + GIG2H (1) Now let R = 0, and cU be the output due to U only: Fig. 2(c).
Consider loop as an open loop. Fig. 2(e).
i. U x G2 x (-GIH) x
U x = GIH x
therefore
(2) 1+GlG2H
Adding the two outputs (equations (1) and (2)).
1+GlG2H 1+GlG2H
Thus
(GIR+U)
Example 17
Determine the output C for the following system. Fig. 2.
H
Figure 2.
Solution
Let UI = U2 = 0 and CR be the output due to R only and, replace the cascaded elements with one element, then system reduces to: Fig. 2(a).
Figure 2(a)
Figure 2(c) Consider loop as an open loop. Fig. 2(c). Then
i. UI - x GIHIH
therefore
CUI _ (2)
Let R — -UI = 0 and cU2 be the output due to I-h only and then system becomes: Fig. 2(d).
Figure 2(d)
Table 2.
The above two tables are from Feedback and Control Systems, by J Distafano 111,
CID
Multi-input Systems - Lecture notes 11
Course: Process Control Systems 3B
University: Durban University of Technology
- Discover more from:
