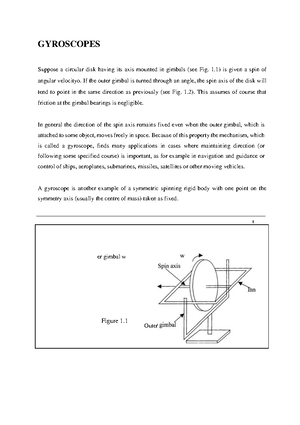
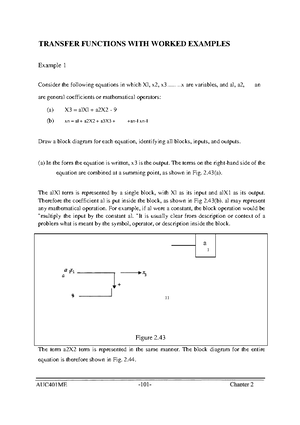
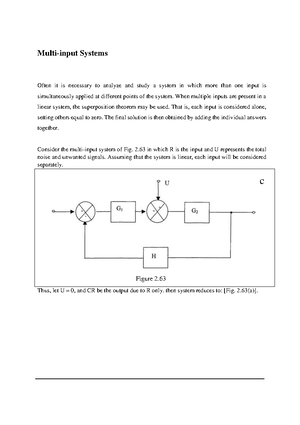
- Information
- AI Chat
Routh'S Stability Criterion
Process Control Systems 3B
Durban University of Technology
Preview text
ROUTH'S STABILITY CRITERION
Open and closed-loop transfer ftmctions have certain basic characteristics that permit transient and steady-state analyses of the feedback-controlled system. Five factors of prime importance in feedback-control systems are stability, the existence and magnitude of the steady-state error, controllability, observatibility, and parameter sensitivity.
The stability characteristic of a linear time-invarient system is determined from the system's characteristic equation. Routh's stability criterion provides a means for determining stability without evaluating the roots of this equation. The steady-state characteristics are obtainable from the open-loop transfer function for unity-feedback systems (or equivalent unity-feedback systems), yielding figures of merit and a ready means for classifying systems.
The response transform X2(s) is given in equation (3), below. Xl(s) is the driving transform.
Section 3 describe the method used to evaluate the inverse transform E l IF(s)] — However, before the inverse transformation can be performed, the polynomial Q(s) must be factored. The stability of the response X2(t) requires that all zeros of Q(s) have negative real parts. Since it is usually not necessary to find the exact solution when the response is unstable, as simple procedure to determine the existence of zeros with real parts is needed.
If such zeros of Q(s) with positive real parts are found, the system the system is unstable and must be modified. Routh's criterion is a simple method of determining the number of zeros with positive real parts without actually solving for the zeros of Q(s). Note that zeros Q(s) are poles of X2(s)
The characteristic equation is:
Q(s) = bnsn + bn-lsn I + bnosn 2 +..... bis + bo
If the bo term is zero, divide by s to obtain the equation in the form of Equation (3). The b's are real coefficients, and all powers of s from sn to s must be present in the characteristic equation. A necessary but not sufficient condition for stable roots is that all the coefficients in Equation (3) must be positive. If any coefficients other than bo are zero, or if all the coefficients do not have the same sign, then there are pure imaginary roots or roots with positive real parts and the system is unstable. In that case it is unnecessary to continue if only stability or instability is to be determined. When all the coefficients are present and positive, the system may or may not be stable because there still may be roots on the imaginary axis or in the right- half s plane. Routh's criterion is mainly used to determine stability. In special
(3)
(3)
c,
This process is continued until no more d terms are present. The rest of the rows are formed in this way down to the s row. The complete array is triangular, ending with the s row. Notice that the s l and s rows contain only one term each. Once the array has been found, Routh's criterion states that the number of roots of the characteristic equation with positive real parts is equal to the number of changes of sign of the coefficients in the first column. Therefore, the system is stable if all terms in the first column have the same sign.
NOTE: The above process is continued until one more row is obtained than the order of
the differential equation. Thus, a third-order equation has four rows, a fourth-order
equation has five rows, etc.
To illustrate, consider the characteristic equation: Q(s) = s 4 + s- 3 + s 2 + 6s + 2 = O
The first two rows of the following array are obtained directly from the coefficients of the characteristic equation, and the remaining rows are computed as just described.
s41 2 0 3 s3 6 s2- 1 2 0 s112 s
- 2;
o ( Third row)
3 3 3
Ixo-
3xo
=
12;
(Fourth row)
-l 1
el = 12
̄ e
12 -o (Fifth row)
and the table cannot be completed
Letting s — , the equation becomes
- 204 +403 +402 = 0 and the array is:
-0,
There are two changes of sign; hence each equation has two roots with positive real parts.
Note: We can solve the problem according to the above method or according to the
procedure applied in Raven.
A Row of Zeros Ref. RAVEN Page 259 Work through this paragraph very carefully and make sure about each step.
Routh'S Stability Criterion
Course: Process Control Systems 3B
University: Durban University of Technology
- Discover more from:
