- Information
- AI Chat
Steady-state error - Lecture notes 17
Process Control Systems 3B
Durban University of Technology
Preview text
Warning: TT: undefined function: 32
Steady-state error
For the designer, it is important to design feedback control systems with minimum error at the output for certain inputs. Techniques for determining system accuracy, are discussed in this paragraph.
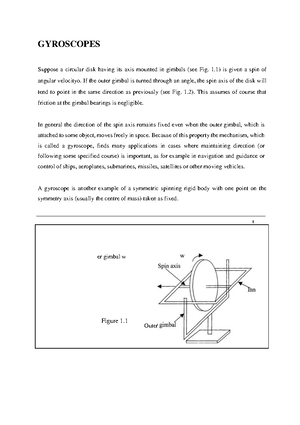
From a theoretical viewpoint, it is desirable for a feedback control system to respond to changes in position (see Fig. C in Appendix C), velocity (see Fig. C) and acceleration (see Fig. C), but this desire is unrealistic and impractical. The requirements for practical systems are fortunately less stringent. For example, consider the automatic positioning system of a missile launcher. It is very important for this type of system to respond, with zero error, to positional-type inputs. A finite tracking error could probably be tolerated for velocity inputs. On the other hand, it is not necessarily important for the system to respond well to acceleration-type inputs.
The final-value theorem of the Laplace transform (see paragraph 3) is used to determine the steady-state performance of any control system. Consider a unity feedback system as shown in Fig. 3.
Figure 3.
The system error, E(s), is given by:
But
Thus
therefore
According to the final-value theorem (paragraph 3), the steady-state error, ess , can be expressed as: sF(s)
— lime(t) = limsE(s) = lim
1 + G(s)
Inputs of position, velocity and acceleration are of interest. Mathematical expressions which represent these physical quantities, are the step, ramp and parabolic functions.
Position input:
1 s
Ramp input:
f(t) = tU(t) 1 S
Acceleration input:
f(t) = t 2 U(t)
1
2
1 + GCs)
= lim I + GCS)
= lim
1
1 + lim G(s)
1 P
with K p lim G(s)
where
KP position constant Table 3 summarises the value of KP for several types of open-loop transfer functions.
Table 3. NUMBER OF PURE INTEGRATIONS OF G(s)
ess
1
1 0
2 0
0
0
k, with k > 0 Table 3 indicates that a unity feedback system with a forward transfer function containing one or more poles at the origin, results in a theoretical steady-state positional response error of zero.
3.4.16 Velocity Constant
Consider the unity feedback system shown in Fig. 3. 1 For a ramp input, f(t) = tU(t) and F(s) = 2 , the steady-state error can be written as S
ess lime(t)
lim sE(s) sF(s) = lim
1
= lim
1 = lim
= lim I + G(s)
1 1
2 0
k, with k > 0 0
3.4.16 Acceleration Constant
Consider the unity feedback system shown in Fig. 3. 1 For a parabolic input, f(t) = t 2 U(t) and F(s) 2 , the steady-state error can be written as
s
= lim e(t) lim sE(s) sF(s) = lim
1
= lim s-»o s2 + s2G(s) 1 = lim s2G(S) because
lims
1
lims2G(s) 1
= lim
with Ka = lims 2 G(s)
where
Ka = acceleration constant
Table 3 summarises the value of Ka for several types of open-loop transfer functions.
Table 3 indicates that a unity feedback system with a forward transfer function containing three or more poles at the origin, results in a theoretical steady-state positional response error of zero.
If the transfer ftmction has zero or one pure integration, the system will not be able to follow a parabolic input. Table 3. NUMBER OF PURE INTEGRATIONS OF G(s)
ess
0
1
2 1
3
0
k, with k > 2
General observation
It seems that the more poles there are at the origin of the complex frequency plane, the more accurate the output will be able to follow the input.
Steady-state error - Lecture notes 17
Course: Process Control Systems 3B
University: Durban University of Technology
- Discover more from:
