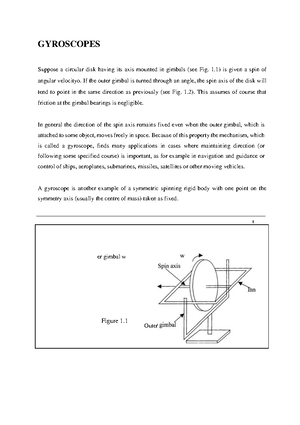
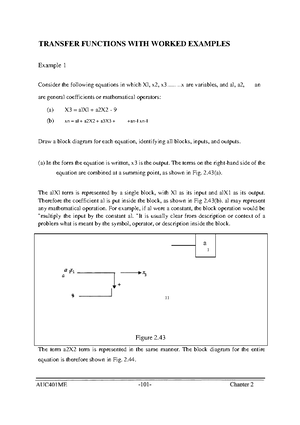
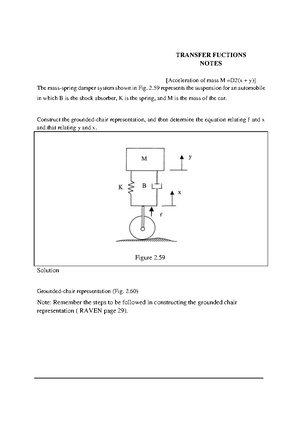
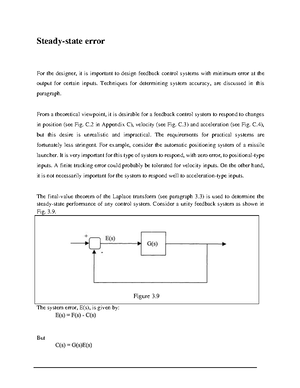
- Information
- AI Chat
Transfer Function - Lecture notes 19
Process Control Systems 3B
Durban University of Technology
Preview text
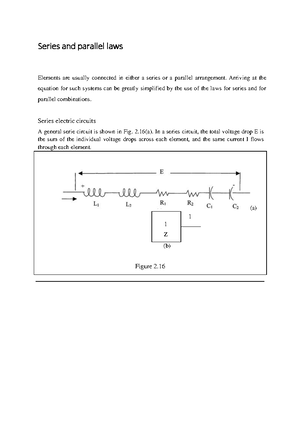
TRANSFER FUNCTION
If the system differential equation is linear, the ratio of the output variable to the input variable, where the variables are expressed as functions of the D operator is called the transfer function.
Consider the system, Fig. 2, where f(t) = [MD 2 + CD + Klx(t)
The system transfer function is: 1 f(t) MD 2 +CD+K (2)
The notation G(D) is used to denote a transfer ftnction when it is expressed in terms of the D operator. It may also be simply as G. The block diagram representation of the system (Fig. 2) represents the mathematical operation G(D) f(t) = x(t); that is the transfer function times the input is equal to the output of the block. The resulting equation is the differential equation of the system.
block diagram representation of Fig 2.
Figure 2.
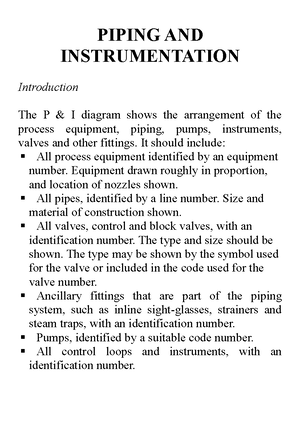
2 MECHANICAL TRANSLATION SYSTEMS
Mechanical systems obey Newton's law that the sum of the forces equals zero; that is, the sum of the applied forces must be equal to the sum of the reactive forces. The three qualities characterising elements in a mechanical translation system are mass, elastance, and damping. The following analysis includes only linear ftmctions. Static friction, Coulomb friction, and other non linear friction terms are not included. Basic elements entailing these qualities are
represented as network elements, and a mechanical network is drawn for each mechanical system to facilitate writing the differential equations.
The mass M is the inertial element. A force applied to a mass produces an acceleration of the mass. The reaction force fM is equal to the product of the mass and acceleration and is opposite in direction to the applied force. In terms of displacement x, velocity v, and acceleration a, the force equation is: 2 fM = Ma = MDv = MDx (2)
The network representation of mass is shown in Fig. 2(a). One tenninal, a, has the motion of the mass; and the other terminal, b, is considered to have the motion of the reference. The reaction force f is a function of time and acts "through" M.
occur unintentionally and is present because of physical construction. The reaction damping force fB is approximated by the product of damping B and the relative velocity of the two ends of the dashpot. The direction of this force, given by Equation (2), depends on the relative magnitudes and directions of the velocities Dxe and DXf: fB = B(ve - Vf) = B(Dxe - DXf) (2)
Damping may be added to a system by use of a dashpot. The basic operation of a dashpot, in which the housing is filled with an incompressible fluid, is shown in Fig. 2. If a force f is applied to the shaft, the piston presses against the fluid, increasing the pressure on side b to side d decreasing the pressure on side a. As a result. the fluid flows around the piston from side b to side a. If necessary, a small hole can be drilled through the piston to provide a positive path for the flow of fluid. The damping force required to move the piston inside the housing is given by Equation (2), where the damping B depends on the dimensions and the fluid used.
Before the differential equations of a complete system can be written, the mechanical network must first be drawn. This is done by connecting the terminals of elements that have the same displacement. Then the force equation is written for each node or position by equating the sum of the forces at each position to zero. The equations are similar to the node equations in an electric circuit, with force analogous to current, velocity analogous to voltage, and the mechanical elements with their appropriate operators analogous to admittance. The reference position in all of the following examples should be taken from the static equilibrium positions. The force of gravity therefore does not appear in the system equations. f(t)
Flled with fluid
Figure 2: Dashpot construction
Simple Mechanical Translation System
The system shown in Fig. 2 is initially at rest. The end of the spring and the mass have positions denoted as the reference positions, and any displacements from these reference
positions are labelled xa and Xb. respectively. A force f applied at the end of the spring must be balanced by a compression of the spring. The same force is also transmitted through the spring and acts at point Xb
To draw the mechanical network, the first step is to locate the points xa and. Xb and the reference. The network elements are then connected between these points. For example, one
end of the spring has the position xa, and the other end has the position Xb. Therefore, the
spring is connected between these points. The complete mechanical network is drawn in Fig.
The displacements xa and Xb are nodes of the circuit. At each node the sum of the forces must add to zero. Accordingly, the equations may be written for nodes a and b as: f = fK=K (xa - (2) fK = fM + fB —- MD 2 + BDXb
(2)
Reference (a) Simple mass- spring- mechanical (b) Corresponding darmer mechanical svstem network
Figure 2.
These two equations can be solved for the two displacements xa and Xb and their respective velocities va = Dxa and Vb = DXb.
It is possible to obtain one equation relating xa to f, Xb to xa, or Xb to f by combining equations (2) and (2):
The multiplication of transfer functions, as in Equation (2), is valid as long as there is no coupling or loading between the two blocks in Fig. 2(a). The signal xa is unaffected by the presence of the block having the transfer function G2, thus the multiplication is valid.
G2(D)
(a) (b)
Figure 2: Block diagram representation of Figure 2: (a) detailed; (b) overall
Multi-Element Mechanical Translation System
A force f(t) is applied to the mass Ml of Fig. 2(a). The sliding friction between the masses Ml and M2 and the surface is indicated by the viscous friction coefficients Bl and B2. The system equations can be written in terms of the two displacements xa and Xb. The mechanical network is drawn by connecting the terminals of the elements that have the same displacement. [See Fig. 2(b)]. Since the sum of the forces at each node must add to zero, the equations are written according to the rules for node equations:
For node a: (MD +BID + B3D + Kl)xa - (B3D)Xb = f (2)
For node b: -(B3D)xa + (M2D 2 + BD + B3D + K2)Xb = O (2) A definite pattern to these equations can be detected. Observe that K 1 , Ml , Bp and B3 are connected to node a and that Equation (2), for node a, contains all four of these terms as coefficients of xa. Notice that element B3 is also connected to node b and that the term -B appears as a coefficient of Xb. When this pattern is used, Equation (2) can be written directly. Thus, since K2, M2, B2, and B3 are connected to node b, they appear as coefficients of Xb. Element B3 is also connected to node a, and -B3 appears as the coefficient of xa. Each term in the equation must be a force.
The node equations for a mechanical system follow directly from the mechanical network of Fig. 2(b).
(a) Multiple-element mechanical system
(b) Corresponding mechanical network
Figure 2.
2 MECHANICAL ROTATIONAL SYSTEMS
The equations characterising rotational systems are similar to those for translation systems. Writing torque equations parallels the writing of force equations, where the displacement, velocity, and acceleration terms are now angular quantities. The applied torque is equal to the sum of the reaction torques. The three elements in a rotational system are inertia, the spring, and the dashpot. The mechanical-network representation of these elements is shown in Fig. 2.
TB = B(oe - Of) = DOC - DOD (2)
Writing the differential equations is simplified by first drawing the mechanical network for the system. This is done by first designating nodes that correspond to each angular displacement. Then each element is connected between the nodes that correspond to the two motions at each end of that element. The inertia elements are connected from the reference node to the node representing its position. The spring and dashpot elements are connected to the two nodes that represent the position of each end of the element. Then the torque equation is written for each node by equating the sum of the torques at each node to zero. These equations are similar to those for mechanical translation and are analogous to those for electric circuits.
Simple Mechanical Rotational Systems
The system shown in Fig. 2 has a mass, with a moment of inertia J, immersed in a fluid. A torque T is applied to the mass. The wire produces a reactive torque proportional to its stiffness K and to the angle of twist. The fins moving through the fluid have a damping B, which requires a torque proportional to the rate at which they are moving. The mechanical network is drawn in Fig. 2. There is one node having displacement 0 ; therefore, only one equation is necessary: JD 2 e + BD9 + = T(t) (2)
Wire
Fluid
Torque
Figure 2.
9
Figure 2.
Multiple-Element Mechanical Rotational System
The system represented by Fig. 2 has two disks that have damping between each of them and the frame. The mechanical network is drawn in Fig. 2 by first identifying the three angular displacements 01, 92 and 03. Then the elements are connected to the proper nodes. The
These three equations can be solved simultaneously for 91, 92 and 93 as a function of the applied torque. If 03 is the system output, these equations can be solved for the following four transfer functions:
91 02 03 G = GIG2G3 — (2) 91 92 1 2
The detailed and overall block diagram representations of Fig. 2 and Fig. 2 are shown in Fig. 2. The overall transfer fimction G given by Equation 2 is the product of the transfer functions, which are said to be in cascade. This product transfer fimctions applies in general to any number of elements in cascade when there is no loading between the blocks.
Note: Transfer functions of systems are explained in the next section
91 92
Transfer Function - Lecture notes 19
Course: Process Control Systems 3B
University: Durban University of Technology

- Discover more from: