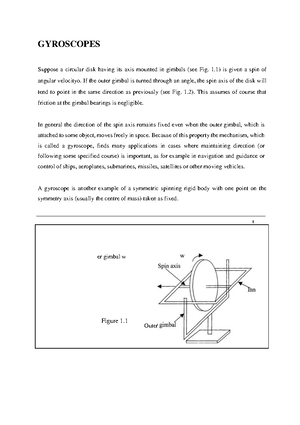
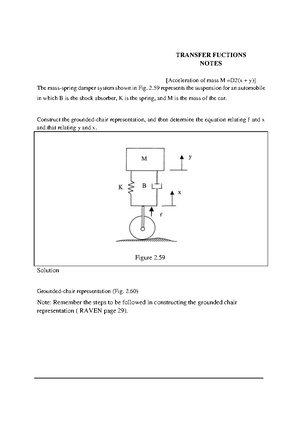
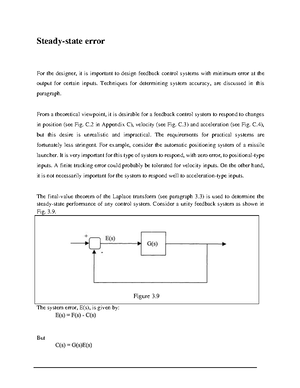
- Information
- AI Chat
Transfer Functions WITH Worked Examples
Process Control Systems 3B
Durban University of Technology
Related Studylists
process controlPreview text
Warning: TT: undefined function: 32
TRANSFER FUNCTIONS WITH WORKED EXAMPLES
Example 1
Consider the following equations in which Xl, x2, x3 x are variables, and al, a2, an are general coefficients or mathematical operators: (a) X3 = alXl + a2X2 - 9 (b) xn = al + a2X2 + a3X3 + +an-l xn-l
Draw a block diagram for each equation, identifying all blocks, inputs, and outputs.
(a) In the form the equation is written, x3 is the output. The terms on the right-hand side of the equation are combined at a summing point, as shown in Fig. 2(a).
The alXl term is represented by a single block, with Xl as its input and alX1 as its output. Therefore the coefficient al is put inside the block, as shown in Fig 2(b). al may represent any mathematical operation. For example, if al were a constant, the block operation would be "multiply the input by the constant al. "It is usually clear from description or context of a problem what is meant by the symbol, operator, or description inside the block.
####### 11
Figure 2.
a
####### 1
The term a2X2 term is represented in the same manner. The block diagram for the entire equation is therefore shown in Fig. 2.
+
####### 9
Following the same line of reasoning as in part (a), the block diagram for xn = alXl + a2X + a3X3 + .........+ an-Ixn-l is shown in Fig. 2.
Figure 2.
Figure 2.
(b) The + and - operations indicate the need for a summing point. The differentiation operation can be treated as in part (a), or by combining two first derivative operations into one second derivative operator block, giving two different block diagrams for the equation for x3, as shown in Fig. 2.
Figure 2.
(c) The integration operation can be represented in block diagram form as shown in Fig. 2.
Figure 2.
3
The system shown in Fig. 2, consisting of a mirror pivoted at one end and adjusted up and down with a screw at the other end. Draw a block diagram for the pivoted, adjustable mirror mechanism. Assume that each 360 0 rotation of the screw raises or lowers the mirror k degrees. Identify all the signals and components of the control system in the diagram.
Figure 250
Solution
The specifications for this problem imply an input equal to the number of rotations of the screw. Let n be the number of rotations of the screw such that n = 0 when 9 = 0 0. Therefore n and 0 can be related by a block diagram described by the constant k, since 0 = kn, as shown in
Fig. 2.
####### — 104 —
Light
Which component or components comprise the plant? The controller? The feedback?
topcock
Figure 2.
Solution
The container is the plant because the water level of the container is being controlled. (See paragraph 24).
The stopper valve may be chosen as the control element; and the ball-float, cord, and associated linkage as the feedback elements. The block diagram is given in Fig, 2.
Figure 2.
The feedback is negative because the water flow rate to the container must decrease as the water level rises in the container.
(d) The system of (c) is closed-loop because the control action (the difference between the volume of traffic in each direction) is a function of the output (actual traffic volume flowing past the intersection in each direction).
(e) Block diagram for the closed-loop traffic signal system.
Figure 2.
NOTE: This system has two outputs, the volume of traffic passing the intersection in one direction (the A direction), and the volume passing the intersection in the other direction (the B direction). The input is the command for equal traffic volumes in directions A and B; that is, the input is zero volume difference.
Suppose we call the mechanism for computing the appropriate red and green timing intervals the Red-Green Time Interval Computer. This device, in addition to the traffic signal, makes
up the control elements. The plants are the roadway in direction A and the roadway in direction B.
6
Ref. RAVEN page 15, example 2. See question in text book.
####### A
####### B
Solution
Cancellation of operators gives 1 1 Df(t) = f(t)
but 1 Df(t) -
therefore
1
Df(t) = f from section 2.
i. 1
f(t) = f fetdtdt — f(et (1)
therefore dy(t)
dt
now if:
y(0)
therefore — CI(O) + C2 ̄ 2
therefore
Y(t) (1) dv du u—dx = uv — f v — dx dx dx (Integration by parts) If
I = 0 -1 + C e 0
therefore 1) The desired result is: y(t) = 2e•t + t - I
Example 9
Ref. RAVEN page 28, example 2. See question in text book.
Solution
Equation relatingfand x The first step is to draw the equivalent grounded-chair system, in which the motion of the mass with respect to ground is clearly indicated, as shown in Fig. 2(b) (Text book).
The spring K is in parallel with the series combination of M and B. Application of the laws for parallel and series elements gives
####### K(MD 2 + BD)
MD 2 + BD + Kx (1)
Equation relatingfand y The force transmitted through the spring K and acts upon the series combination of M and B.
####### (MD + BD)
Thus the equation of motion for this part of the system which relates f and y is
(MD 2 + BD) y (2)
3. Equation relating x and y
The desired relationship between x and y is obtained by eliminating f between equations (1) and (2).
Solution
- Force equation at each co-ordinate
x
Figure 2.
####### (1)
(KI + - y) -K2Y = MD 2 y (2)
therefore
(Kl +1<2 +BD+MD
####### 2
####### 2 (3)
(Kl +BD K 2 +MD
(b) Equation which relate y tof
y(K2 + MD 2 ) = f
therefore 1
(4)
(c) Equation which relate y to x
The desired relationship between y and x is obtained by eliminating f between equations (3) and (4).
therefore
x (5)
2 Equation relating x to fby means ofthe grounded-chair method
Solution
] The first step is to draw the equivalent grounded-chair system, in which the motion of the mass with respect to ground is clearly indicated, as shown in Fig. 2.
Figure 2.
The series combination of spring Kl and B is in parallel with the series combination of K2 and M. Application of the laws for parallel and series elements gives
d2x A schematic diagram of an accelerometer for measuring the linear acceleration is shown dt in Fig. 2. Determine the operational form for the differential equation which relates y (the change in the position of the mass relative to the frame) to the acceleration D 2 x of the frame.
Solution
Restoring force = accelerating force 2 i. -Ky BDy = MD(x + y) 2 = MD 2 x i. -MDy - Ky BDy i. y(MD 2 + K + BD) = -MD 2 x
therefore - MD 2 x MD 2 + BD+K
Transfer Functions WITH Worked Examples
Course: Process Control Systems 3B
University: Durban University of Technology

- Discover more from: