- Information
- AI Chat
AVL Tree - AVL TREE DATA STRUCTURE FULL NOTES WITH FIGURE
Btech (kcs-701)
APJ Abdul Kalam Technological University
Recommended for you
Related Studylists
Data structure 💥Preview text
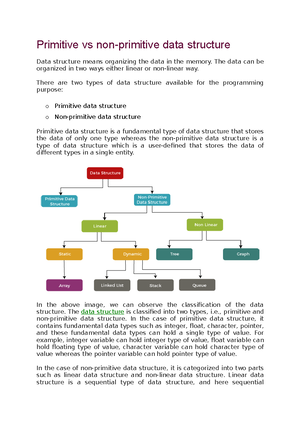
AVL Tree
AVL Tree is invented by GM Adelson - Velsky and EM Landis in 1962. The tree is named AVL in honour of its inventors.
AVL Tree can be defined as height balanced binary search tree in which each node is associated with a balance factor which is calculated by subtracting the height of its right sub-tree from that of its left sub-tree.
Tree is said to be balanced if balance factor of each node is in between - to 1, otherwise, the tree will be unbalanced and need to be balanced.
Balance Factor (k) = height (left(k)) - height (right(k))
If balance factor of any node is 1, it means that the left sub-tree is one level higher than the right sub-tree.
33 724 Exception Handling in Java - Javatpoint
If balance factor of any node is 0, it means that the left sub-tree and right sub-tree contain equal height.
If balance factor of any node is -1, it means that the left sub-tree is one level lower than the right sub-tree.
An AVL tree is given in the following figure. We can see that, balance factor associated with each node is in between -1 and +1. therefore, it is an example of AVL tree.
Complexity
Algorithm Average case Worst case
Space o(n) o(n)
Search o(log n) o(log n)
Insert o(log n) o(log n)
Delete o(log n) o(log n)
Operations on AVL tree
Due to the fact that, AVL tree is also a binary search tree therefore, all the operations are performed in the same way as they are performed in a binary search tree. Searching and traversing do not lead to the violation in
When BST becomes unbalanced, due to a node is inserted into the right subtree of the right subtree of A, then we perform RR rotation, RR rotation is an anticlockwise rotation, which is applied on the edge below a node having balance factor -
In above example, node A has balance factor -2 because a node C is inserted in the right subtree of A right subtree. We perform the RR rotation on the edge below A.
2. LL Rotation
When BST becomes unbalanced, due to a node is inserted into the left subtree of the left subtree of C, then we perform LL rotation, LL rotation is clockwise rotation, which is applied on the edge below a node having balance factor 2.
In above example, node C has balance factor 2 because a node A is inserted in the left subtree of C left subtree. We perform the LL rotation on the edge below A.
3. LR Rotation
Double rotations are bit tougher than single rotation which has already explained above. LR rotation = RR rotation + LL rotation, i., first RR rotation is performed on subtree and then LL rotation is performed on full tree, by full tree we mean the first node from the path of inserted node whose balance factor is other than -1, 0, or 1.
Let us understand each and every step very clearly:
State Action
A node B has been inserted into the right subtree of A the left subtr of C, because of which C has become an unbalanced node havi balance factor 2. This case is L R rotation where: Inserted node is in the right subtree of left subtree of C
As LR rotation = RR + LL rotation, hence RR (anticlockwise) on subtr rooted at A is performed first. By doing RR rotation, node A , become the left subtree of B.
After performing RR rotation, node C is still unbalanced, i., havi balance factor 2, as inserted node A is in the left of left of C
Now we perform LL clockwise rotation on full tree, i. on node node C has now become the right subtree of node B, A is left subtre B
Balance factor of each node is now either -1, 0, or 1, i. BST balanced now.
4. RL Rotation
Balance factor of each node is now either -1, 0, or 1, i., BST balanced now.
Q: Construct an AVL tree having the following elements
H, I, J, B, A, E, C, F, D, G, K, L
1. Insert H, I, J
On inserting the above elements, especially in the case of H, the BST becomes unbalanced as the Balance Factor of H is -2. Since the BST is right-skewed, we will perform RR Rotation on node H.
The resultant balance tree is:
2. Insert B, A
On inserting the above elements, especially in case of A, the BST becomes unbalanced as the Balance Factor of H and I is 2, we consider the first node from the last inserted node i. H. Since the BST from H is left- skewed, we will perform LL Rotation on node H.
The resultant balance tree is:
3 a) We first perform RR rotation on node B
The resultant tree after RR rotation is:
3b) We first perform LL rotation on the node I
The resultant balanced tree after LL rotation is:
4. Insert C, F, D
On inserting C, F, D, BST becomes unbalanced as the Balance Factor of B and H is -2, since if we travel from D to B we find that it is inserted in the right subtree of left subtree of B, we will perform RL Rotation on node I. RL = LL + RR rotation.
4a) We first perform LL rotation on node E
The resultant tree after LL rotation is:
On inserting G, BST become unbalanced as the Balance Factor of H is 2, since if we travel from G to H, we find that it is inserted in the left subtree of right subtree of H, we will perform LR Rotation on node I. LR = RR + LL rotation.
5 a) We first perform RR rotation on node C
The resultant tree after RR rotation is:
5 b) We then perform LL rotation on node H
The resultant balanced tree after LL rotation is:
6. Insert K
On inserting K, BST becomes unbalanced as the Balance Factor of I is -2. Since the BST is right-skewed from I to K, hence we will perform RR Rotation on the node I.
The resultant balanced tree after RR rotation is:
AVL Tree - AVL TREE DATA STRUCTURE FULL NOTES WITH FIGURE
Course: Btech (kcs-701)
University: APJ Abdul Kalam Technological University
This is a preview
Access to all documents
Get Unlimited Downloads
Improve your grades
