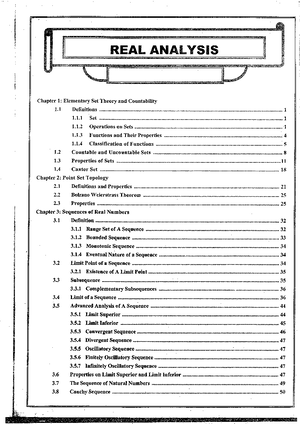
- Information
- AI Chat
1527147289 E-textof Chapter 2Module 1
Mathematics
Sikkim Manipal University
Recommended for you
Students also viewed
- 18MA41A
- Sonya Jain(11-A) Maths Practical File
- DPP-4 Non abelian Groups
- TC 002Part No 001 SUP 2 - Ok gds ghj
- 1 100 merged 29 12 2022 - Ibsihuabinsin
- Trisem 252022-23 STS5003 SS VL2022230300003 Reference Material I 31-10-2022 Fallsem 2021-22 STS5001 SS VL202122010 5252 Reference Material I 13-09-2021 Business Etiquette
Related documents
Preview text
CHAPTER 2. VECTOR SPACES
MODULE 1. INTRODUCTION TO VECTOR SPACES
INDRANATH SENGUPTA
Contents
Fields 1
Vector spaces over an arbitrary field 2
Fields
Definition 1. A binary operation on a non empty set X is a function ◦ : X × X → X. For every x, y ∈ X, the element ◦(x, y) is a well defined element in X, denoted by x ◦ y.
Example 1. The usual addition and multiplication are examples of binary operations on the sets Z, Q, R and C.
Example 1.
α 1 .. . αn
+
β 1 .. . βn
=
α 1 + β 1 .. . αn + βn
defines a binary operation on Rn(respectively Cn). This is known as pointwise addition of n-tuples.
Definition 2. Let F be a nonempty set with two binary operations + and · called addition and multiplication on F. We call (F, +, ·) a field if (F, +) and (F \ { 0 }, ·) are abelian groups and the following distributive property holds :
x.(y + z) = x + x ∀x, y, z ∈ F.
The additive identity of (F, +) is denoted by 0.
The multiplicative identity of (F \ { 0 }, ·) is denoted by 1.
1
2 Module 1
Example 1. (Q, +, ·), (R, +, ·), (C, +, ·) are examples of infinite fields, where + and · are the usual addition and multiplication respec- tively.
Example 1. (Zp, +, ·) is a finite field, where p is a prime number and +, · are the addition and multiplication of integers modulo p re- spectively.
Example 1. Q[
√
2] = {a + b
√
2 |a, b ∈ Q} is a field, where the binary operations are defined as
(a + b
√
- (c + d
√
- = (a + c) + (b + d)
√
2
and (a + b
√
- · (c + d
√
- = (ac + 2bd) + (ad + bc)
√
2 ,
where a, b, c, d ∈ Q.
- Vector spaces over an arbitrary field
Definition 3. Let (V, +) be an abelian group. Let F be a field. Sup- pose that there exists a scalar multiplication F × V → V , written as (λ, x) → λ.x. The abelian group (V, +) is said to be a vector space over the field F if
(i) λ.(x + y) = λ.x + λ.y for all λ ∈ F and x, y ∈ V ; (ii) (λ + μ).x = λ.x + μ.x for all λ, μ ∈ F and x ∈ V ; (iii) (λμ).x = λ.(μ.x) for all λ, μ ∈ F and x ∈ V ; (iv) 1 = x for all x ∈ V.
Let 0 denote the additive identity of the vector space V. Recall that 0 and 1 denote the additive identity and the multiplicative identity of the field F respectively.
Elements of the vector space V are called vectors in V and elements of the field F are called scalars in F.
Example 2. Rn and Cn are two basic models for (finite dimensional ) vector spaces over the fields R and C respectively.
Example 2. In general, Fn is a vector space over the field F.
4 Module 1
Theorem 2. Let V be a vector space over a field F. Let 0 and 0 denote the additive identities of V and F respectively.
(i) 0 = 0 for all x ∈ V. (ii) (−1).x = −x x ∈ V , where −x is the additive inverse of x in V. (iii) α. 0 = 0 for all α ∈ F. (iv) If α.x = 0 for α ∈ F and x ∈ V , then either α = 0 or x = 0.
Proof. (i) 0 = (0 + 0).x = 0 + 0; therefore, 0 = 0.
(ii) 1 + (−1).x = (1 + (−1)).x = 0 = 0. (iii) α. 0 = α.( 0 + 0 ) = α. 0 + α. 0 ; therefore, α. 0 = 0. (iv) If α 6 = 0 then α− 1 exists in F. Moreover, α.x = 0 implies that α− 1 .(α.x) = α− 1. 0 = 0. Therefore, x = 1 = (α− 1 .α).x = α− 1 .(α.x) = 0.
1527147289 E-textof Chapter 2Module 1
Course: Mathematics
University: Sikkim Manipal University
- More from:MathematicsSikkim Manipal University666 Documents
Recommended for you
Students also viewed
- 18MA41A
- Sonya Jain(11-A) Maths Practical File
- DPP-4 Non abelian Groups
- TC 002Part No 001 SUP 2 - Ok gds ghj
- 1 100 merged 29 12 2022 - Ibsihuabinsin
- Trisem 252022-23 STS5003 SS VL2022230300003 Reference Material I 31-10-2022 Fallsem 2021-22 STS5001 SS VL202122010 5252 Reference Material I 13-09-2021 Business Etiquette